Received 19 July 2016; accepted 3 September 2016; published 6 September 2016

ABSTRACT

By using fluid dynamics theory with the effects of adsorption and reaction, the chromatography model with a reaction A ® B was established as a system of two hyperbolic partial differential equations (PDE’s). In some practical situations, the reaction chromatography model was simplified a semi-coupled system of two linear hyperbolic PDE’s. In which, the reactant concentration wave model was the initial-boundary value problem of a self-closed hyperbolic PDE, while the resultant concentration wave model was the initial-boundary value problem of hyperbolic PDE coupling reactant concentration. The general explicit expressions for the concentration wave of the reactants and resultants were derived by Laplace transform. The d-pulse and wide pulse injections were taken as the examples to discuss detailedly, and then the stability analysis between the resultant solutions of the two modes of pulse injection was further discussed. It was significant for further analysis of chromatography, optimizing chromatographic separation, determining the physical and chemical characters.

Keywords:

Reaction Chromatography Model, Hyperbolic Partial Differential Equations, Initial-Boundary Problem, Stability Analysis

1. Introduction

With the appearance of diverse production chromatography (such as the reaction chromatography), the chromato- graphy technology has been widely applied in chemistry, chemical engineering, biological engineering and pharmaceutical engineering, etc., while the demand of chromatography theory is increasing higher. The relation- ships among the chromatographic input-output and the system conditions play the very important role in chromatography model [1] - [6] .

In fact, the mathematical model of chromatography system is a initial-boundary value problem of hyperbolic partial differential equations system [7] - [11] , which is hard and challenging mathematics problem to chromato- graphy scientists. In the other hand, the practical application and demand for chromatography is also difficult to understand deeply by mathematicians. The relative works of partial differential equations in the practical chromatography are still not enough.

If the chromatographic process contains reactions, it is labeled as reaction chromatography. An important example is the catalyst for the column packing, accompanied the catalytic [2] - [6] in the adsorption process, and the isomerization reaction is the common situation.

In this paper, a chromatography model with a reaction 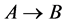was established, which is a initial-boundary value problem for the semi-coupled system of two linear hyperbolic partial differential equations. Then the general explicit expressions of concentration waves for reactant and resultant were derived using Laplace transform. It was significant for further analysis between input and output of chromatography, optimizing chromatographic separation, determining the physical and chemical characters. Finally, the d-pulse and wide pulse injections were taken as the examples to discuss detailedly, and then the stability analysis between the resultant solutions of the two modes of pulse injection was further discussed. The results provided proper theory models for further chromatographic data analysis.

2. Reaction Chromatography Model

Set the concentrations of the reactant A and the resultant B in the mobile phase and in the stationary phase as 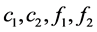respectively. Reaction rate was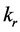. And the linear velocity of the mobile phase was u. The volume shares in chromatographic column in the mobile phase and in the stationary phase as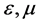, respectively.

Denoted that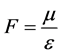, then the mass conservation equations between reactant and resultant in the catalytic

chromatographic process was shown as below:

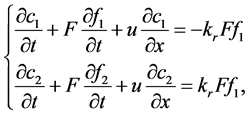(1)

where, 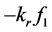was the reactant reduction rate, and 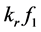was resultant increase rate, 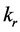was the coefficient of reaction rate. According to Langmuir type adsorption isotherms, 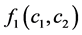and 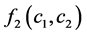satisfied for:

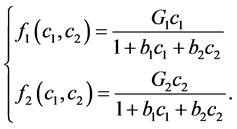(2)

The concentration wave Equation (1) were a system of two nonlinear hyperbolic partial differential equations, which was a hard mathematical problem. But in some practical situations, the problem can be simplified [2] . Assume 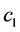was small, or the adsorption coefficient 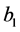was small, that was,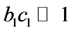. While considering the assumed reaction rate is relatively minor, then was also small, that was, ,. In fact, in the quantitative analysis using high performance liquid chromatography (HPLC), the concentrations of most analytes, such as the reactant A and the resultant B here, were all very small [2] [3] . Thus the adsorption isotherm above can be approximated as a linear and regarded as follows:

(3)

and denoted concretely:

(4)

they were positive constant, thus Equation (1) can be simplified to the following semi-coupled system of two linear hyperbolic partial differential equations. In which, the reactant concentration wave model was the initial- boundary value problem of a self-closed hyperbolic partial differential equations, while the resultant con- centration wave model was the initial boundary value problem of hyperbolic partial differential equations coupling reactant concentration.

(5)

Chromatographic process started from the boundary, and there were many types of the boundary conditions, such as the injection methods of d-pulse, wide pulse, head-on, etc.; whose corresponding boundary condition were not zero. The initial state of chromatography columns were typically empty, that the initial conditions corresponding to 0. However, in practical problems, there were some important chromatograph whose corre- sponding initial conditions is not zero, such as simulated moving bed chromatography. Therefore, it is necessary to study the general initial-boundary value problem with both the initial and boundary values were not 0. That was, satisfied the following the general initial-boundary value problems.

(6)

(7)

where, were constants, were positive piecewise and continuous smooth functions, and meet the compatibility condition,.

3. Explicit Solution of Concentration Wave

Firstly, solved the initial-boundary value problem (6) for concentration wave of of reactant. According to Laplace transform of t, noted that:

it follows from (6) that

(8)

Then solved the ordinary differential Equation (8) about, we got:

and

Since

(9)

and

That is to say,

(10)

To sum (9) and (10) up,

(11)

Then solved the initial-boundary value problem (7) for the concentration wave of resultant. Similarly, according to Laplace transform of t, noted that:

The above problem (7) satisfied the following ordinary differential equation:

(12)

Solved the ordinary differential Equation (12) about, we got:

Hence, we got

Since

(13)

and

where

(14)

Meanwhile, we had

and

(15)

To sum (13), (14) and (15) up,

(16)

Using the expression (11) of and the relation Equation (16) of and, the explicit solution ex- pressions of were derived by dividing into the following three cases.

In the case of, we got

(17)

In the case of, set, then we had

(18)

In the case of, we had, then we got

(19)

Particularly, when the initial-boundary problem (6) and (7) satisfied the following conditions

the explicit solution of reactant and resultant concentration wave were obtained as follows.

Following (11), we had

(20)

According to the expressions (17), (18) and (19), we had the explicit solution expressions of as follows.

When

(21)

When

(22)

When

(23)

4. Solutions and Stability for d-Pulse and Wide Pulse Injections

In this section, we derived the solutions of reactant and resultant concentration waves in wide pulse and d-pulse injections detailedly. And the stability analysis between the resultant solutions of the two modes of pulse injection was further discussed.

4.1. d-Pulse Injection

Chromatographic process started from the boundary, and there were many types of the boundary conditions, such as the methods of d-pulse, wide pulse, head-on, etc; whose corresponding boundary condition was not zero. Where, d-pulse and wide pulse were the most common way of chromatography injection method. Firstly, initial state of chromatography column in the d-pulse method, which injection function was a kind of d-function, was typically empty. So in the case of d-Pulse, satisfied the following initial-boundary problem.

(24)

where k is a constant represented the injection size, which is equal to in wide pulse method in Section 4.2. According to the behavior of the d-function, we had

The solution of concentration wave for reactant was obtained by Laplace transform as similar with Section 3. The concentration wave corresponding to d-pulse injection of reactant and resultant can be expressed as follows.

(25)

If there was no reaction terms, that was, , we got

(26)

As for the solution of concentration wave for resultant, the initial and boundary values were both 0. From the expression (21), (22) and (23), we had the explicit solution expressions of.

When

(27)

It was equivalent to

When

(28)

When

(29)

4.2. Wide Pulse Injection

Wide pulse was the another most common way of chromatography injection method, its initial state of chromato- graphy column was typically empty, so the initial condition was the follows,

The corresponding injection function was given as follows,

(30)

where, was the injection time, was the injection rate, both of them are constant. In this paper, Wide pulse was taken as an another example, the solution of concentration wave for reactant and resultant were derived detailedly.

Similarly, we had the explicit solution expressions of and as follows,

(31)

When,

(32)

When, we got,

(33)

When,

(34)

4.3. Stability Analysis between Wide Pulse and d-Pulse Injections

Note that, the boundary condition in wide pulse injection tended to the condition in d-pulse injection. We also showed that the mentioned limit relationship was still valid for the solutions in the two modes of pulse injection. The main result of this work is the following theorem:

Theorem 1. If and, the solution of concentration wave for resultant in wide pulse injection converges to the resultant solution in d-pulse injection.

Proof. 1) When, from (32), For any fixed, when, we had

and when, (a sufficiently small constant), so that, and

By arbitrariness of, we obtained

(35)

which was converging to the solution (27) in d-pulse injection.

2) When, the resultant solution (33) in wide pulse injection can be expressed as follows.

In the case of,

(36)

In the case of,

(37)

For any fixed, (a sufficiently small constant), so that. Then expressions (37) can be

noted to:

(38)

Furthermore, for any fixed,

a) When, we had

b) When, (a sufficiently small constant), , we got

and

c) When, (a sufficiently small constant), , we had

To sum up,

(39)

3) When, the solution in wide pulse method (34) was equivalent to the following.

In the case of,

(40)

In the case of,

(41)

For any fixed, (a sufficiently small constant), so that. Then expression (41) can be

noted to:

(42)

and for any,

i) When, we had

ii) When, (a sufficiently small constant), , we had

iii) When, (a sufficiently small constant), , we had

By arbitrariness of and, we obtained

(43)

By (35), (39) and (43), we can conclude that this Theorem is true. □

5. Conclusion

The chromatography model with a reaction was established and can be simplified a semi-coupled system of two linear hyperbolic PDE's in some practical situations. In which, the reactant concentration wave model was the initial-boundary value problem of a self-closed hyperbolic PDE, while the resultant concentration wave model was the initial-boundary value problem of hyperbolic PDE coupling reactant concentration. The general explicit expressions for the concentration wave of the reactants and resultants were derived by Laplace transform. The d-pulse and wide pulse injections were taken as the examples to discuss detailedly, and it was proved that the continuous dependence of solutions was in accordance with the dependence under corresponding boundary conditions. It was significant for further analysis of chromatography in nonlinear case, optimizing chromatographic separation, determining the physical and chemical characters.

Acknowledgements

This work was supported by National Natural Science Foundation of China (No. 21312045) and Science and Technology Project of Guangdong Province of China (No. 2014A020213016).

Cite this paper

Jing Zhang,Maofei Shao,Tao Pan, (2016) Concentration Wave for a Class of Reaction Chromatography System with Pulse Injections. American Journal of Computational Mathematics,06,224-236. doi: 10.4236/ajcm.2016.63023

References

NOTES

^*Corresponding author.