Application of OHAM-DJ to the System of Burgers’ Equations
Vol.06No.03(2016), Article ID:69675,12 pages
10.4236/ajcm.2016.63022
Fatheah Ahmad Alhendi1, Bothayna Saleh Kashkari1, Aisha Abdullah Alderremy2
1Department of Mathematics, King Abdulaziz University, Jeddah, Saudi Arabia
2Department of Mathematics, King Khalid University, Abha, Saudi Arabia

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 31 May 2016; accepted 8 August 2016; published 11 August 2016
ABSTRACT
In this paper, the system of Burgers’ equations is solved by the optimal homotopy asymptotic method with Daftardar-Jafari polynomials OHAM-DJ. Two numerical examples are illustrated the efficient of this methods for solving the system of Burgers’ equations.
Keywords:
Burgers’ Equations, The Optimal Homotopy Asymptotic Method, Daftardar-Jafari Polynomials

1. Introduction
The Burgers equation was first presented by Bateman [1] and treated later by J. M. Burgers (1895-1981) then it is widely named as Burgers’ equation [2] . Burgers’ equation is nonlinear partial differential equation of second order which is used in various fields of physical phenomena such as boundary layer behaviour, shock weave formation, turbulence, the weather problem, mass transport, traffic flow and acoustic transmission [3] [4] . In addition, the two dimentional Burgers’ equations have played an important role in many physical applications such as investigating the shallow water waves and modeling of gas dynamics [5] [6] . In order to a great applications for burgers’ equations many researchers have been interested in solving it by various techniques. Analytic solution of one dimensional Burgers’ equation are get by many standard methods such as Backland transformation method, differential transformation method and tanh-coth method [6] , while an analytical solution of two dimensional Burgers’ equations was first presented by Fletcher using the Hopf-Cole transfor- mation [7] . The finite difference, finite element, spectral methods, Adomian decomposition method, the varia- tional iteration method, homotopy perturbation method HPM and Eulerian-Lagrangian method gave an nu- merical solution of Burgers’ equations [3] [8] - [15] .
Recently, the OHAM was proposed by Marinca and Herisaun [16] - [19] . OHAM is independent of the existence of a embedding parameter in the problem then overcome the limitations of perturbation technique. However, OHAM is the most generalized form of HPM as it uses a general auxiliary function H(p). This method has been studied by a number of researchers for solving linear and nonlinear partial differential equations [20] - [23] . In [24] - [27] proved OHAM is more efficient to solve Burgers’ equations. In 2006, a new method by Daftardar-Gejji and Jafari for solving nonlinear functional appeared [28] . Convergence of it has been proved in [29] . This method is named later as Daftardar-Jafari method DJM in [30] . J. Ali et al. used DJM in the OHAM for solving non-linear differential equations and they named this method as OHAM with DJ polynomials OHAM-DJ [30] [31] . In 2016, OHAM-DJ has been used to solve linear and nonlinear Klein-Gordon equations [32] . The motive of this paper is to show the efficiency of OHAM-DJ for solving the system of Burger’s equations. We consider the system of Burger’s equations as the following [11] :
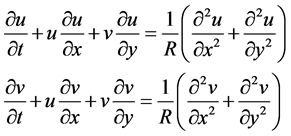 (1.1)
(1.1)
with the initial conditions:
 (1.2)
(1.2)
and the boundary conditions:
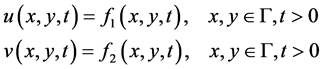 (1.3)
(1.3)
where 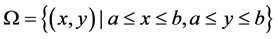 and
and 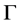 is its boundary,
is its boundary, 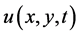 and
and 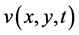 are the velocity components to be determined,
are the velocity components to be determined, 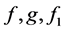 and
and 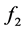 are known functions and R is the Reynolds number.
are known functions and R is the Reynolds number.
This paper is organized into three sections. In Section 2 methodology of OHAM-DJ is presented. In Section 3 application of this method is solved and absolute error of approximate solutions of proposed method is com- pared with exact solutions. In all cases the proposed method yields better results.
2. Methodology of OHAM-DJ
Consider (1.1) and let
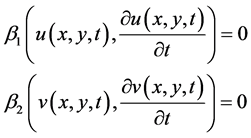 (2.1)
(2.1)
where 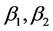 are boundary operators.
are boundary operators.
According to the basic idea of OHAM [16] , we can construct the optimal homotopy:
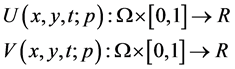 (2.2)
(2.2)
which satisfies
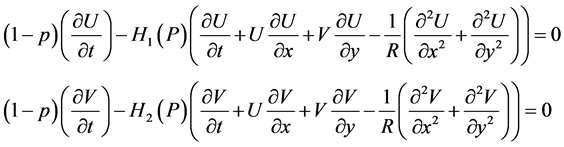 (2.3)
(2.3)
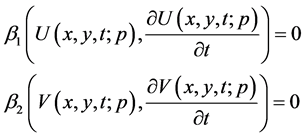 (2.4)
(2.4)
where 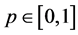 is an embedding parameter while
is an embedding parameter while  and
and  is initial approximation of Equation (1.1) which satisfies the boundary condition,
is initial approximation of Equation (1.1) which satisfies the boundary condition,  and
and  are nonzero auxiliary functions for
are nonzero auxiliary functions for ,
, and
and . Clearly, When
. Clearly, When  and
and , it holds that
, it holds that ,
,  and
and ,
,  respectively. Therefore, as p change from 0 to 1, the solution
respectively. Therefore, as p change from 0 to 1, the solution  and
and  varies from
varies from  to
to  and
and  to
to  respectively, where the initial approximations
respectively, where the initial approximations  and
and  are obtained from (2.3) and (2.4). Now, choosing The auxiliary functions
are obtained from (2.3) and (2.4). Now, choosing The auxiliary functions  and
and  as the form
as the form
 (2.5)
(2.5)
where,  are constants to be determined later. Assume that the solutions of (1.1) has the form:
are constants to be determined later. Assume that the solutions of (1.1) has the form:
 (2.6)
(2.6)
The nonlinear terms
 (2.7)
(2.7)
are decomposed as
 (2.8)
(2.8)
where  are (DJ) polynomials,
are (DJ) polynomials,
 . For simplicity these polynomials are expressed as:
. For simplicity these polynomials are expressed as:

Then,
 (2.9)
(2.9)
Substiting, (2.5),(2.6), (2.7) and (2.9) into (2.3), and comparing the coefficients of like powers of p, we get
 (2.10)
(2.10)
The convergence of (2.6) depend upon the auxiliary constants  and
and , which known convergence control parameters or optimal convergence control parameters [16] , if it is convergent at
, which known convergence control parameters or optimal convergence control parameters [16] , if it is convergent at  we have
we have
 (2.11)
(2.11)
Substituting (2.11) into (1.1) we get the residuals  and
and ,
,  these parameters can be optimal identified by various methods [16] [20] [33] . Optimization method is one of theses methods to find out the optimal convergence control parameters by means of the minimum of the squared residuals.
these parameters can be optimal identified by various methods [16] [20] [33] . Optimization method is one of theses methods to find out the optimal convergence control parameters by means of the minimum of the squared residuals.
3. Numerical Examples
In this section, two numerical examples are used to prove the efficiency and the accuracy of the method which we proposed for the system of Burgers’ equations.
3.1. Example 1
Consider the system of two dimensional of Burgers’ equations with the initial conditions as following [34]
 (3.1)
(3.1)
with the initial conditions:
 (3.2)
(3.2)
The exact solutions are
 (3.3)
(3.3)
Accordance to the methodology of OHAM-DJ, 
 (3.4)
(3.4)
Their solutions are
 (3.5)
(3.5)
Then,
 (3.6)
(3.6)
By substituting (3.6) into (3.1) we get the residuals and using the optimization method we have computed that  and
and . Finally, putting the values of
. Finally, putting the values of  and
and  into (3.6), to get the approximate solutions (Tables 1-3, Figure 1 and Figure 2).
into (3.6), to get the approximate solutions (Tables 1-3, Figure 1 and Figure 2).

Figure 1. Approximation solutions by OHAM-DJ of example 1, t = 0.01, .
.

Figure 2. Exact solutions of example 1, t = 0.01, .
.
Table 1. Comparison of OHAM-DJ solutions with exact solutions at mesh point x = 2, y = 1 (example 1).
3.2. Example 2
We consider the following two-dimensional Burgers’ equations [34]
 (3.7)
(3.7)
On square domain , with the initial condition:
, with the initial condition:
 (3.8)
(3.8)
for which the exact solution is . Where the
. Where the  and
and 
in (1.1) are symmetry in this example,  and the initial condition are symmetry also.
and the initial condition are symmetry also.
Table 2. Comparison of OHAM-DJ solutions with exact solutions at mesh point x = 1, y = 2 (example 1).
Table 3. Comparison of OHAM-DJ solutions with exact solutions at mesh point x = 1.5, y = 2 (example 1).
 (3.9)
(3.9)
Their solutions are
 (3.10)
(3.10)
 (3.11)
(3.11)
Substituting (3.11) into (3.7) we get the residuals and using the optimization method we have computed that . Finally, putting the values of
. Finally, putting the values of  into (3.11) to get the approximate solutions (Table 4 and Table 5, Figure 3 and Figure 4).
into (3.11) to get the approximate solutions (Table 4 and Table 5, Figure 3 and Figure 4).
4. Conclusion
In this work, the OHAM-DJ is applied to obtain numerical solutions of the system of Burgers’ equations. The method is efficient and easy to implement where the first or second order solutions rapidly converges to the exact solutions. Furthermore, OHAM-DJ does not need any discretization in time or in space. Thus the solutions of system of Burgers’ equations are not influenced by computer round off errors. The method can be easily
Table 4. Comparison of OHAM-DJ solutions with exact solutions at mesh point x = 1, y = 1,  (example 2).
(example 2).
Table 5. Comparison of OHAM-DJ solutions with exact solutions at mesh point x = 1, y = 1.5,  (example 2).
(example 2).

Figure 3. Approximation solutions by OHAM-DJ of example 2, t = 0.01, .
.

Figure 4. Exact solutions of example 2, t = 0.01, .
.
extended to other nonlinear equations. Nutshell, OHAM-DJ is a better numerical method for solving nonlinear equations.
Acknowledgements
This paper was funded by King Abdulaziz City for Science and Technology (KACST) in Saudi Arabia. The authors therefore, thank them for their full collaboration.
Cite this paper
Fatheah Ahmad Alhendi,Bothayna Saleh Kashkari,Aisha Abdullah Alderremy, (2016) Application of OHAM-DJ to the System of Burgers’ Equations. American Journal of Computational Mathematics,06,212-223. doi: 10.4236/ajcm.2016.63022
References
- 1. Shah, Z., Nawaz, R., Shah, S., Shah, S.I.A. and Shah, M. (2016) Use of the Daftardar-Jafari Poly-Nomials in Optimal Homotopy Asymptotic Method for the Solution of Linear and Nonlinear Klein-Gordon Equations. Journal of Applied Environmental and Biological Sciences, 6, 71-81.
- 2. Pathak, S.P. and Singh, T. (2015) Solution of Coupled Non-Linear System by Optimal Homotopy Analysis Method. International Journal of Conceptions on Computing and Information Technology, 3, 49.
- 3. Yu, X., Zhao, G. and Zhang, R. (2011) The New Numerical Method for Solving the System of Two-Dimensional Burgers’ Equations. Computers & Mathematics with Applications, 62, 3279-3291.
http://dx.doi.org/10.1016/j.camwa.2011.08.044 - 4. Ali, J., Shah, S., Islam, S. and Khan, H. (2013) Application of Optimal Homotopy Asymtotic Method with Daftardar-Jaffari Polynomials to Non-Linear Differential Equations. World Applied Sciences Journal, 28, 1456-1462.
- 5. Ali, J. (2013) Analysis and Application of Optimal Homotopy Asymptotic Method and the Use of Daftardar Jaffery Polynomials. Doctoral Dissertation, Islamia College Peshawar, Peshawar.
- 6. Bhalekar, S. and Daftardar-Gejji, V. (2011) Convergence of the New Iterative Method. International Journal of Differential Equations, 2011, Article ID: 989065.
http://dx.doi.org/10.1155/2011/989065 - 7. Daftardar-Gejji, V. and Jafari, H. (2006) An Iterative Method for Solving Nonlinear Functional Equations. Journal of Mathematical Analysis and Applications, 316, 753-763.
http://dx.doi.org/10.1016/j.jmaa.2005.05.009 - 8. Islam, S., Nawaz, R., Arif, M. and Shah, I.A. (2012) Application of Optimal Homotopy Asymptotic Method to the Equal Width Wave and Burger Equations. Life Science Journal, 9, 2380-2386.
- 9. Jafari, H. and Gharbavy, S. (2012) The Solution of KDV-Burgers Equation by the Optimal Homotopy Asymptotic Method. Studies in Nonlinear Sciences, 3, 1-7.
- 10. Ishaq, M. and Haq, S. (2014) An Efficient Numerical Method for the Solution of Coupled Two Dimensional Burger’s Equations. World Applied Sciences Journal, 29, 277-286.
- 11. Nawaz, R., Ullah, H., Islam, S. and Idrees, M. (2013) Application of Optimal Homotopy Asymptotic Method to Burger Equations. Journal of Applied Mathematics, 2013, Article ID: 387478.
http://dx.doi.org/10.1155/2013/387478 - 12. Iqbal, S., Idrees, M., Siddiqui, A.M. and Ansari, A.R. (2010) Some Solutions of the Linear and Nonlinear Klein-Gordon Equations Using the Optimal Homotopy Asymptotic Method. Applied Mathematics and Computation, 216, 2898-2909.
http://dx.doi.org/10.1016/j.amc.2010.04.001 - 13. Ullah, H., Nawaz, R., Islam, S., Idrees, M. and Fiza, M. (2015) The Optimal Homotopy Asymptotic Method with Application to Modified Kawahara Equation. Journal of the Association of Arab Universities for Basic and Applied Sciences, 18, 82-88.
http://dx.doi.org/10.1016/j.jaubas.2014.05.004 - 14. Kashkari, B.S. (2014) Application of Optimal Homotopy Asymptotic Method for the Approximate Solution of Kawahara Equation. Applied Mathematical Sciences, 8, 875-884.
- 15. Gupta, A.K. and Ray, S.S. (2014) Comparison between Homotopy Perturbation Method and Optimal Homotopy Asymptotic Method for the Soliton Solutions of Boussinesq-Burger Equations. Computers & Fluids, 103, 34-41.
http://dx.doi.org/10.1016/j.compfluid.2014.07.008 - 16. Marinca, V. and Herisanu, N. (2008) Application of Optimal Homotopy Asymptotic Method for Solving Nonlinear Equations Arising in Heat Transfer. International Communications in Heat and Mass Transfer, 35, 710-715.
http://dx.doi.org/10.1016/j.icheatmasstransfer.2008.02.010 - 17. Marinca, V., Herisanu, N., Bota, C. and Marinca, B. (2009) An Optimal Homotopy Asymptotic Method Applied to the Steady Flow of a Fourth-Grade Fluid Past a Porous Plate. Applied Mathematics Letters, 22, 245-251.
http://dx.doi.org/10.1016/j.aml.2008.03.019 - 18. Marinca, V., Herisanu, N. and Nemes, I. (2008) Optimal Homotopy Asymptotic Method with Application to Thin Film Flow. Open Physics, 6, 648-653.
http://dx.doi.org/10.2478/s11534-008-0061-x - 19. Marinca, V. and Herisanu, N. (2015) The Optimal Homotopy Asymptotic Method: Engineering Applications. Springer International Publishing, Gewerbestrasse.
http://dx.doi.org/10.1007/978-3-319-15374-2 - 20. Young, D.L., Fan, C.M., Hu, S.P. and Atluri, S.N. (2008) The Eulerian-Lagrangian Method of Fundamental Solutions for Two-Dimensional Unsteady Burgers’ Equations. Engineering Analysis with Boundary Elements, 32, 395-412.
http://dx.doi.org/10.1016/j.enganabound.2007.08.011 - 21. Desai, K.R. and Pradhan, V.H. (2012) Solution of Burger’s Equation and Coupled Burger’s Equations by Homotopy Perturbation Method. International Journal of Engineering Research and Applications (IJERA), 2, 2033-2040.
- 22. M.A. Abdou and A.A. Soliman. Variational iteration method for solving burger's and coupled burger's equations. Journal of Computational and Applied Mathematics, 181(2):245-251, 2005.
http://dx.doi.org/10.1016/j.cam.2004.11.032 - 23. Ahmad, J., Bibi, Z. and Noor, K. (2014) Laplace Decomposition Method Using He’s Polynomial to Burgers Equation. Journal of Science and Arts, 14, 131.
- 24. Khan, M. (2014) A Novel Solution Technique for Two Dimensional Burger’s Equation. Alexandria Engineering Journal, 53, 485-490.
http://dx.doi.org/10.1016/j.aej.2014.01.004 - 25. Mittal, R.C. and Sighal, P. (1996) Numerical Solution of Periodic Burgers Equation. Indian Journal of Pure and Applied Mathematics, 27, 689-700.
- 26. Kutluay, S., Bahadir, A.R. and Ozdes, A. (1999) Numerical Solution of One-Dimensional Burgers Equation: Explicit and Exact-Explicit Finite Difference Methods. Journal of Computational and Applied Mathematics, 103, 251-261.
http://dx.doi.org/10.1016/S0377-0427(98)00261-1 - 27. Fletcher, C.A.J. (1983) A Comparison of Finite Element and Finite Difference Solutions of the One- and Two-Dimensional Burgers’ Equations. Journal of Computational Physics, 51, 159-188.
http://dx.doi.org/10.1016/0021-9991(83)90085-2 - 28. Fletcher, C.A. (1983) Generating Exact Solutions of the Two-Dimensional Burgers’ Equations. International Journal for Numerical Methods in Fluids, 3, 213-216.
http://dx.doi.org/10.1002/fld.1650030302 - 29. Srivastava, V.K., Ashutosh and Tamsir, M. (2013) Generating Exact Solution of Three Dimensional Coupled Unsteady Nonlinear Generalized Viscous Burgers’ Equations. International Journal of Mathematical Sciences, 5, 1-13.
- 30. Logan, J.D. (1994) An Introduction to Nonlinear Partial Differential Equations. Wily-Interscience, Balkema.
- 31. Naghipour, A. and Manafian, J. (2015) Application of the Laplace Adomian Decomposition and Implicit Methods for Solving Burgers’ Equation. TWMS Journal of Pure and Applied Mathematics, 6, 68-77.
- 32. Wazwaz, A.M. (2002) Partial Differential Equations: Methods and Applications.
- 33. Burgers, J.M. (1948) A Mathematical Model Illustrating the Theory of Turbulence. Advances in Applied Mechanics, 1, 171-199.
http://dx.doi.org/10.1016/S0065-2156(08)70100-5 - 34. Bateman, H. (1915) Some Recent Researches on the Motion of Fluids. Monthly Weather Review, 43, 163-170.
http://dx.doi.org/10.1175/1520-0493(1915)43<163:SRROTM>2.0.CO;2
上一篇:On the Location of Zeros of Po 下一篇:An Optimal Policy with Quadrat
最新文章NEWS
- Auto-Bäcklund Transformation and Extended Tanh-Function Methods to Solve the Time-Dependent Coeffici
- A Third-Order Scheme for Numerical Fluxes to Guarantee Non-Negative Coefficients for Advection-Diffu
- Conjugate Effects of Radiation and Joule Heating on Magnetohydrodynamic Free Convection Flow along a
- An O(k<sup>2</sup>+kh<sup>2</sup>+h<sup>2</sup>) Accurate Two-le
- On the Location of Zeros of Polynomials
- Peristaltic Pumping of a Conducting Sisko Fluid through Porous Medium with Heat and Mass Transfer
- An Accurate Numerical Integrator for the Solution of Black Scholes Financial Model Equation
- Simulation of Time-Dependent Schrödinger Equation in the Position and Momentum Domains
推荐期刊Tui Jian
- Chinese Journal of Integrative Medicine
- Journal of Genetics and Genomics
- Journal of Bionic Engineering
- Pedosphere
- Chinese Journal of Structural Chemistry
- Nuclear Science and Techniques
- 《传媒》
- 《哈尔滨师范大学自然科学学报》
热点文章HOT
- Asymptotic Solutions for the Fifth Order Critically Damped Nonlinear Systems in the Case for Small E
- Partial Fraction Decomposition by Repeated Synthetic Division
- Higher-Order Numerical Solution of Two-Dimensional Coupled Burgers’ Equations
- Group Method Analysis of MHD Mixed Convective Flow Past on a Moving Curved Surface with Suction
- Simple and Multi Linear Regression Model of Verbs in Quran
- Peristaltic Pumping of a Conducting Sisko Fluid through Porous Medium with Heat and Mass Transfer
- Conjugate Effects of Radiation and Joule Heating on Magnetohydrodynamic Free Convection Flow along a
- An O(k<sup>2</sup>+kh<sup>2</sup>+h<sup>2</sup>) Accurate Two-le

