Received 16 July 2015; accepted 6 September 2015; published 9 September 2015

ABSTRACT

Adomian decomposition method is presented as a method for the solution of the Burger’s equation, a popular PDE model in the fluid mechanics. The method is computationally simple in application. The approximate solution is obtained by considering only the first two terms of the decomposition in this paper. Numerical experimentation shows accuracy of a minimum error of order five for various space steps and coefficient of kinematic viscosity. The method is considered high in accuracy.

Keywords:

Adomian Decomposition, Burger’s Equation, Kinematic Viscosity, Nonlinear Operators

1. Introduction

Burger’s equation is a fundamental partial differential equation in fluid mechanics. It is also a very important model encountered in several areas of applied mathematics such as heat conduction, acoustic waves, gas dynamics and traffic flow [1] . Analytical solutions of the partial differential equations modeling physical phenomena exist only in few of the cases. Therefore the need for the construction of efficient numerical methods for the approximate solution of these models always exists. Many of the analytical solutions to the Burger’s equation involve Fourier series. According to [2] , the convergence of such Fourier-series based solutions is very slow. Several researchers have proposed various numerical methods for the solution of the Burger’s equation. [3] and [4] used the finite element method for the solution of the Burger’s equation. [5] and [6] used the finite difference method. [7] used the direct variational method while [8] used the projection method by B-spline.

A decomposition method which provides convergent solutions to nonlinear stochastic operator equations was developed in [9] . [10] gave proof of convergence of Adomian decomposition method when applied to differential equations. [11] constructed an algorithm for solving nonlinear equations based on Newton-Raphson method using Adomian decomposition approach. In an effort to extend the usefulness of Adomian’s decomposition method into other areas of mathematics, [12] developed a technique for calculating Adomian’s polynomials for nonlinear operators. [13] presented a method for the solution of homogeneous differential equations using Adomian’s decomposition without noisy terms. [14] extended the application of the Adomian decomposition method for the calculations of non-differential functions.

In this paper, presentation of a numerical method for the solution of the nonlinear reaction-diffusion Burger’s equation using Adomian decomposition is made. The organization of this paper is as follows: in Section 2 the theoretical approach is presented. In Section 3 the Adomian’s polynomials for the Burger’s equation are determined. In Section 4 computational results for the Burger’s equation using Adomian’s decomposition are presented while conclusion is presented in Section 5.

2. Theoretical Approach

Consider the Burger’s equation

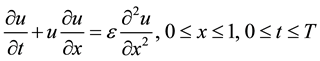(1)

Subject to initial condition

(2)

And boundary conditions:

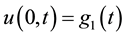(3)

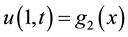(4)

Defining the operators:

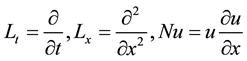(5)

Equation (1) can be written as:

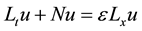(6)

Solving Equation (6) for 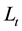and respectively gives

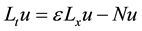(7)

and

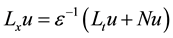(8)

where and 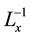are the inverse operators of 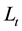and 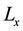given respectively as:

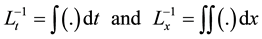(9)

Operating both sides of Equations (7) and (8) with the inverse operators (9) we obtain:

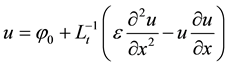(10)

(11)

where

(12)

and

(13)

Adding Equations (10) and (11) and dividing by 2, gives the canonical form:

(14)

The parameterized form of (14) is

(15)

where

(16)

According to [15] the parameterized decomposition forms of and N_u are:

(17)

(18)

A_n are the Adomian’s polynomials which can be generated for all types of nonlinearities [16] .

Substituting Equations (17) and (18) into Equation (15) gives

(19)

Expanding both sides of Equation (19) gives

(20)

By comparing coefficients of both hand sides of Equation (20), it is obtained that:

From which we establish the recursive relation

(21)

3. Determination of Adomian’s Polynomials

The Adomian’s polynomials, are defined in such a way that

[17]

Substituting Equation (17) into Equation (18) and expanding the RHS gives

(22)

From Equation (22), we establish that the Adomian’s polynomials have the following forms:

The Adomian’s polynomials for Equation (1) are obtained from the recurrent relation

(23)

4. Numerical Experiment

We present numerical results to illustrate the effectiveness of the proposed method. Consider Burger’s Equation (1) with the following initial and boundary conditions.

The exact solution of Equation (1) with the above conditions was given in [1] as

Computations of Absolute and Relative Errors

Absolute errors of the method were computed by use of the formula:

where the numerical solution at the grid point is, and the exact solution at the same grid point is.

Relative errors were computed by use of the formula:

where the numerical solution at the grid point is, and the exact solution at the same grid point is.

Figure 1 is a 3 dimensional plot of the surface while Figure 2 is a 3 dimensional plot of the surface

5. Conclusion

In this paper, Adomian decomposition method is used to solve the burger’s equation numerically. From Table 1 and Table 2, this method is considered to give accurate results for specified values of the parameter, ε. We considered the first two terms of the decomposition to make the approximate solution. Accuracy of the method

can be improved by considering more terms in the solution approximation. All computations were carried out using Maple 15.

Cite this paper

Iyakino P.Akpan, (2015) Adomian Decomposition Approach to the Solution of the Burger’s Equation. American Journal of Computational Mathematics,05,329-335. doi: 10.4236/ajcm.2015.53030

References