On Existence of Solutions of q-Perturbed Quadratic Integral Equations
Vol.06No.02(2016), Article ID:67794,11 pages
10.4236/ajcm.2016.62018
Maryam Al-Yami
Department of Mathematics, Al Faisaliah Campus, Sciences Faculty, King Abdulaziz University, Jeddah, Saudi Arabia

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 18 April 2016; accepted 26 June 2016; published 29 June 2016
ABSTRACT
We investigate a q-fractional integral equation with supremum and prove an existence theorem for it. We will prove that our q-integral equation has a solution in 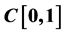 which is monotonic on
which is monotonic on . The monotonicity measures of noncompactness due to Banaś and Olszowy and Darbo’s theorem are the main tools used in the proof of our main result.
. The monotonicity measures of noncompactness due to Banaś and Olszowy and Darbo’s theorem are the main tools used in the proof of our main result.
Keywords:
q-Fractional, Integral Equation, Monotonic Solutions, Darbo Theorem, Monotonicity Measure of Noncompactness

1. Introduction
Jackson in [1] introduced the concept of quantum calculus (q-calculus). This area of research has rich history and several applications, see [2] - [4] and references therein. There are several developments and applications of the q-calculus in mathematical physics, especially concerning quantum mechanics, the theory of relativity and special functions [5] [4] . Recently, several researchers attracted their attention by the concept of q-calculus, and we could find several new results in [6] [7] and the references therein.
In several papers among them [8] - [11] , integral equations with nonsigular kernels have been studied. In [12] - [14] Darwish et al. introduced and studied the quadratic Volterra equations with supremum. Also, Banaś et al. and Darwish [13] [15] - [17] studied quadratic integral equations of arbitrary orders with singular kernels. In [18] , Darwish generalized and extended Banaś et al. [15] results to the perturbed quadratic integral equations of arbitrary orders with singular kernels.
In this paper, we will study the q-perturbed quadratic integral equation with supremum
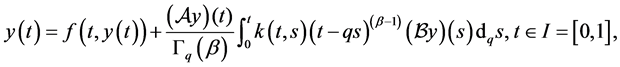 (1)
(1)
where ,
, 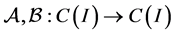 , and
, and .
.
By using Darbo fixed point theorem and the monotonicity measure of noncompactness due to Banaś and Olszowy [19] , we prove the existence of monotonic solution to Equation (1) in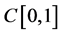 .
.
2. q-Calculus and Measure of Noncompactness
First, we collect basic definitions and results of the q-fractional integrals and q-derivatives, for more details, see [5] [6] [20] [21] and references therein.
First, for a real parameter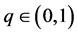 , we define a q-real number
, we define a q-real number  by
by
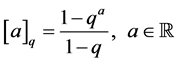 , (2)
, (2)
and a q-analog of the Pochhammer symbol (q-shifted factorial) is defined by
 (3)
(3)
Also, the q-analog of the power 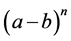 is given by
is given by
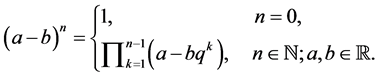 (4)
(4)
Moreover,
 (5)
(5)
Notice that,  exists and we will denote it by
exists and we will denote it by .
.
More generally, for  we define
we define
 (6)
(6)
and
 (7)
(7)
Notice that . Therefore, if
. Therefore, if , then
, then .
.
The q-gamma function is defined by
 (8)
(8)
where  Or, equivalently,
Or, equivalently,  and satisfies
and satisfies 
Next, the q-derivative of a function f is given by
 (9)
(9)
and the q-derivative of higher order of a function f is defined by
 (10)
(10)
The q-integral of a function f defined on the interval  is defined by
is defined by
 (11)
(11)
If f is given on the interval  and
and  then
then
 (12)
(12)
The operator  is defined by
is defined by
 (13)
(13)
The fundamental theorem of calculus satisfies for  and
and , i.e.,
, i.e., , and if f is continuous at
, and if f is continuous at , then
, then .
.
The following four formulas will be used later in this paper
 (14)
(14)
and
 (15)
(15)
where  denotes the q-derivative with respect to variable t.
denotes the q-derivative with respect to variable t.
Notice that, if  and
and , then
, then .
.
Definition 1. [2] Let f be a function defined on . The fractional q-integral of the Riemann-Liouville type of order
. The fractional q-integral of the Riemann-Liouville type of order  is given by
is given by
 (16)
(16)
Notice that, for , the above q-integral reduces to (11).
, the above q-integral reduces to (11).
Definition 2. [2] The fractional q-derivative of the Riemann-Liouville type of order  is given by
is given by
 (17)
(17)
where  denotes the smallest integer greater than or equal to
denotes the smallest integer greater than or equal to .
.
In q-calculus, the derivative rule for the product of two functions and integration by parts formulas are
 (18)
(18)
Lemma 1. Let  and f be a function defined on
and f be a function defined on . Then the following formulas are verified:
. Then the following formulas are verified:
 (19)
(19)
Lemma 2. [21] For , using q-integration by parts, we have
, using q-integration by parts, we have
 (20)
(20)
or
 (21)
(21)
Second, we recall the basic concepts which we need throughout the paper about measure of noncompactness.
We assume that 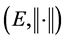 is a real Banach space with zero element
is a real Banach space with zero element  and we denote by
and we denote by  the closed ball with radius r and centered x, where
the closed ball with radius r and centered x, where .
.
Now, let  and denote by
and denote by  and Conv X the closure and convex closure of X, respectively. Also, the symbols
and Conv X the closure and convex closure of X, respectively. Also, the symbols  and
and  stands for the usual algebraic operators on sets.
stands for the usual algebraic operators on sets.
Moreover, the families  and
and  are defined by
are defined by  and
and  respectively.
respectively.
Definition 3. [22] Let  If the following conditions
If the following conditions
1) .
.
2) 
3) 
4)  and
and
5) if  is a sequence of closed subsets of
is a sequence of closed subsets of  with
with  and
and 
then  hold. Then, the mapping
hold. Then, the mapping  is said to be a measure of noncompactness in E.
is said to be a measure of noncompactness in E.
Here,  is the kernel of the measure of noncompactness
is the kernel of the measure of noncompactness .
.
Our result will establish in C(I) the Banach space of all defined, continuous and real functions on  with
with .
.
Next, we defined the measure of noncompactness related to monotonicity in , see [19] [22] .
, see [19] [22] .
We fix a bounded subset  of
of . For
. For  and
and  denotes the modulus of continuity of the function y given by
denotes the modulus of continuity of the function y given by
 . (22)
. (22)
Moreover, we let
 (23)
(23)
and
 (24)
(24)
Define
 (25)
(25)
and
 (26)
(26)
Notice that, all functions in Y are nondecreasing on I if and only if .
.
Now, we define the map  on
on  as
as
 (27)
(27)
Clearly, μ verifies all conditions in Definition 3 and, therefore it is a measure of noncompactness in  [19] .
[19] .
Definition 4.Let  Let
Let  be a continuous operator. Suppose that
be a continuous operator. Suppose that  maps bounded sets onto bounded ones. If there exists a bounded
maps bounded sets onto bounded ones. If there exists a bounded  with
with , then
, then  is said to be satisfies the Darbo condition with respect to a measure of noncompactness
is said to be satisfies the Darbo condition with respect to a measure of noncompactness .
.
If , then
, then  is called a contraction operator with respect to
is called a contraction operator with respect to .
.
Theorem 1. [23] Let  be a bounded, convex and closed subset of E. If
be a bounded, convex and closed subset of E. If  is a Contraction operator with respect to
is a Contraction operator with respect to . Then
. Then  has at least one fixed point belongs to Q.
has at least one fixed point belongs to Q.
3. Existence Theorem
Let us consider the following suggestions:
a1)  is continuous and
is continuous and

Moreover,  and
and 
a2) The superposition operator F generated by the function f satisfies for any nonnegative function y the condition , where c is the same constant as in a1).
, where c is the same constant as in a1).
a3)  is a continuous operator which satisfies the Darbo condition for the measure of noncompactness
is a continuous operator which satisfies the Darbo condition for the measure of noncompactness  with a constant
with a constant . Also,
. Also,  if
if .
.
a4) .
.
a5) The function  is continuous on
is continuous on  and nondecreasing
and nondecreasing  and separately. Moreo-
and separately. Moreo-
ver, 
a6)  is a continuous operator and there is a nondecreasing function
is a continuous operator and there is a nondecreasing function  such that
such that  for any
for any . Moreover, for every function
. Moreover, for every function  which is nonnegative on I, the function
which is nonnegative on I, the function  is nonnegative and nondecreasing on I.
is nonnegative and nondecreasing on I.
a7)  such that
such that
 (28)
(28)
and .
.
Before, we state and prove our main theorem, we define the two operators  and
and  on
on  as follows
as follows
 (29)
(29)
and
 (30)
(30)
respectively. Finding a fixed point of the operator  defined on the space
defined on the space  is equivalent to solving Equation (1).
is equivalent to solving Equation (1).
Theorem 2. Assume the suggestions (a1)-(a7) be verified, then Equation (1) has at least one solution  which is nondecreasing on I.
which is nondecreasing on I.
Proof. We divide the proof into seven steps for better readability.
Step 1: We will show that the operator  maps
maps  into itself.
into itself.
For this, it is sufficient to show that  if
if . Fix
. Fix  and let
and let  and
and  with
with . We have
. We have
 (31)
(31)
Notice that, we have used
 (32)
(32)
Notice that, since the function k is uniformly continuous on , then when
, then when  we have that
we have that .
.
Thus , and therefore,
, and therefore, 
Step 2:  applies
applies  into itself.
into itself.
Now,  , we have
, we have
 (33)
(33)
Hence
 (34)
(34)
Therefore, if  we get from assumption a7) the following
we get from assumption a7) the following
 (35)
(35)
Therefore,  maps
maps  into itself.
into itself.
We define the subset  of
of  by
by
 (36)
(36)
It is clear that  is closed, convex and bounded.
is closed, convex and bounded.
Step 3:  applies the set
applies the set  into itself.
into itself.
By this facts and suggestions a1), a4) and a6), we obtain  transforms
transforms  into itself.
into itself.
Step 4: The operator  is continuous on
is continuous on .
.
To prove this, we fix  to be a sequence in
to be a sequence in  with
with . We will show that
. We will show that .
.
Thus, we have ,
,
 (37)
(37)
Consequently,
 (38)
(38)
As  and
and  are continuous operators,
are continuous operators,  such that
such that
 (39)
(39)
Also,  such that
such that
 (40)
(40)
Furthermore,  such that
such that
 (41)
(41)
Now, take , then (38) gives us that
, then (38) gives us that
 . (42)
. (42)
This shows that  is continuous in
is continuous in .
.
Step 5: In recognition of  with respect to the quantity
with respect to the quantity .
.
Now, we take  Let us fix an arbitrarily number
Let us fix an arbitrarily number  and choose
and choose  and
and  with
with . We will be supposed that
. We will be supposed that  because no generality will be loss. Then, by using our suggestions and inequality (31), we get
because no generality will be loss. Then, by using our suggestions and inequality (31), we get
 (43)
(43)
The last estimate implies
 (44)
(44)
and, consequently,
 (45)
(45)
Since the function k is uniformly continuous on  and the function f is continuous on
and the function f is continuous on , then the last inequality gives us that
, then the last inequality gives us that
 (46)
(46)
Step 6: In recognition of  with respect to the quantity d.
with respect to the quantity d.
Here, we fix an arbitrary  and
and  with
with . Then, by our assumption, we obtain our suggestions, we have
. Then, by our assumption, we obtain our suggestions, we have
 (47)
(47)
Now, we will prove that
 (48)
(48)
We find that
 (49)
(49)
But,  because
because  is increasing with respect to t, then
is increasing with respect to t, then
 (50)
(50)
and, since  is negative for
is negative for  then
then
 (51)
(51)
Inequalities (50) and (51) imply that

This inequality and (47) gives us
 (52)
(52)
The above estimate implies that
 (53)
(53)
Therefore,
 (54)
(54)
Step 7:  is contraction with respect to the measure of noncompactness
is contraction with respect to the measure of noncompactness .
.
Inequalities (46) and (54) give us that
 (55)
(55)
or
 (56)
(56)
But , then
, then
 (57)
(57)
Inequality (57) enables us to use Theorem 1, then there are solutions to Equation (1) in .
.
This finishes our proof.
Cite this paper
Maryam Al-Yami, (2016) On Existence of Solutions of q-Perturbed Quadratic Integral Equations. American Journal of Computational Mathematics,06,166-176. doi: 10.4236/ajcm.2016.62018
References
- 1. Jackson, F.H. (1910) On q-Definite Integrals. The Quarterly Journal of Pure and Applied Mathematics, 41, 193-203.
- 2. Agarwal, R.P. (1969) Certain Fractional q-Integrals and q-Derivatives. Proceedings of the Cambridge Philosophical Society, 66, 365-370.
http://dx.doi.org/10.1017/S0305004100045060 - 3. Jleli, M., Mursaleen, M. and Samet, B. (2016) Q-Integral Equations of Fractional Orders. Electronic Journal of Differential Equations, 2016, 1-14.
http://dx.doi.org/10.1186/s13662-015-0739-5 - 4. Kac, V. and Cheung, P. (2002) Quantum Calculus. Springer-Verlag, New York.
http://dx.doi.org/10.1007/978-1-4613-0071-7 - 5. Annaby, M.H. and Mansour, Z.S. (2012) q-Fractional Calculus and Equations. Lecture Notes in Mathematics, Springer, Heidelberg, 2056.
- 6. Abdeljawad, T. and Baleanu, D. (2011) Caputo q-Fractional Initial Value Problems and a q-Analogue Mittag-Leffler Function. Communications in Nonlinear Science and Numerical Simulation, 16, 4682-4688.
http://dx.doi.org/10.1016/j.cnsns.2011.01.026 - 7. Salahshour, S., Ahmadian, A. and Chan, C.S. (2015) Successive Approximation Method for Caputo q-Fractional IVPs. Communications in Nonlinear Science and Numerical Simulation, 24, 153-158.
http://dx.doi.org/10.1016/j.cnsns.2014.12.014 - 8. Banaś, J., Lecko, M. and El-Sayed, W.G. (1998) Existence Theorems of Some Quadratic Integralequations. Journal of Mathematical Analysis and Applications, 222, 276-285.
http://dx.doi.org/10.1006/jmaa.1998.5941 - 9. Banaś, J., Caballero, J., Rocha, J. and Sadarangani, K. (2005) Monotonic Solutions of a Class of Quadratic Integral Equations of Volterra Type. Computers & Mathematics with Applications, 49, 943-952.
http://dx.doi.org/10.1016/j.camwa.2003.11.001 - 10. Caballero, J., Lopez, B. and Sadarangani, K. (2005) On Monotonic Solutions of an Integral Equation of Volterra Type with Supremum. ournal of Mathematical Analysis and Applications, 305, 304-315.
http://dx.doi.org/10.1016/j.jmaa.2004.11.054 - 11. Darwish, M.A. (2007) On Solvability of Some Quadratic Functional-Integral Equation in Banach Algebra. Communications in Applied Analysis, 11, 441-450.
- 12. Darwish, M.A. (2007) On a Singular Quadratic Integral Equation of Volterra Type with Supremum. IC/2007/071, Trieste, Italy, 1-13.
- 13. Darwish, M.A. (2008) On Existence and Asympototic Behaviour of Solutions of a Fractional Integral Equation with Linear Modification of the Argument. arXiv: 0805.1422v1.
- 14. Darwish, M.A. (2008) On Monotonic Solutions of a Singular Quadratic Integral Equation with Supremum. Dynamic Systems and Applications, 17, 539-550.
- 15. Banaś, J. and Rzepka, B. (2007) Monotonic Solutions of a Quadratic Integral Equation of Fractional Order. Journal of Mathematical Analysis and Applications, 332, 1370-1378.
- 16. Banaś, J. and O’Regan, D. (2008) On Existence and Local Attractivity of Solutions of a Quadratic Integral Equation of Fractional Order. Journal of Mathematical Analysis and Applications, 345, 573-582.
http://dx.doi.org/10.1016/j.jmaa.2008.04.050 - 17. Darwish, M.A. (2005) On Quadratic Integral Equation of Fractional Orders. ournal of Mathematical Analysis and Applications, 311, 112-119.
http://dx.doi.org/10.1016/j.jmaa.2005.02.012 - 18. Darwish, M.A. and Ntouyas, S.K. (2009) Monotonic Solutions of a Perturbed Quadratic Fractional Integral Equation, Nonlinear Analysis: Theory. Methods and Applications, 71, 5513-5521.
- 19. Banaś, J. and Olszowy, L. (2001) Measures of Noncompactness Related to Monotonicity. Commentationes Mathematicae, 41, 13-23.
- 20. Bhaskar, T.G., Lakshmikantham, V. and Leela, S. (2009) Fractional Differential Equations with a Krasnoselskii-Krein Type Condition. Nonlinear Analysis: Hybrid Systems, 3, 734-737.
http://dx.doi.org/10.1016/j.nahs.2009.06.010 - 21. Rajković, P.M., Stanković, S.D. and Miomir, S. (2007) Fractional Integrals and Derivatives in q-Calculus. Applicable Analysis and Discrete Mathematics, 1, 311-323.
http://dx.doi.org/10.2298/AADM0701311R - 22. Banaś, J. and Goebel, K. (1980) Measures of Noncompactness in Banach Spaces. Lecture Notes in Pure and Applied Mathematics. Marcel Dekker, New York, 60.
- 23. Dugundji, J. and Granas, A. (1982) Fixed Point Theory. Monografie Mathematyczne, PWN, Warsaw.
上一篇:On the Location of Zeros of Po 下一篇:Partial Fraction Decomposition
最新文章NEWS
- Auto-Bäcklund Transformation and Extended Tanh-Function Methods to Solve the Time-Dependent Coeffici
- A Third-Order Scheme for Numerical Fluxes to Guarantee Non-Negative Coefficients for Advection-Diffu
- Conjugate Effects of Radiation and Joule Heating on Magnetohydrodynamic Free Convection Flow along a
- An O(k<sup>2</sup>+kh<sup>2</sup>+h<sup>2</sup>) Accurate Two-le
- On the Location of Zeros of Polynomials
- Peristaltic Pumping of a Conducting Sisko Fluid through Porous Medium with Heat and Mass Transfer
- An Accurate Numerical Integrator for the Solution of Black Scholes Financial Model Equation
- Simulation of Time-Dependent Schrödinger Equation in the Position and Momentum Domains
推荐期刊Tui Jian
- Chinese Journal of Integrative Medicine
- Journal of Genetics and Genomics
- Journal of Bionic Engineering
- Pedosphere
- Chinese Journal of Structural Chemistry
- Nuclear Science and Techniques
- 《传媒》
- 《哈尔滨师范大学自然科学学报》
热点文章HOT
- Asymptotic Solutions for the Fifth Order Critically Damped Nonlinear Systems in the Case for Small E
- Partial Fraction Decomposition by Repeated Synthetic Division
- Higher-Order Numerical Solution of Two-Dimensional Coupled Burgers’ Equations
- Group Method Analysis of MHD Mixed Convective Flow Past on a Moving Curved Surface with Suction
- Simple and Multi Linear Regression Model of Verbs in Quran
- Peristaltic Pumping of a Conducting Sisko Fluid through Porous Medium with Heat and Mass Transfer
- Conjugate Effects of Radiation and Joule Heating on Magnetohydrodynamic Free Convection Flow along a
- An O(k<sup>2</sup>+kh<sup>2</sup>+h<sup>2</sup>) Accurate Two-le
