Comparison between Adomian’s Decomposition Method and Toeplitz Matrix Method for Solving Linear Mixe
Vol.06No.02(2016), Article ID:67897,7 pages
10.4236/ajcm.2016.62019
Fatheah Ahmed Hendi1, Manal Mohamed Al-Qarni2
1Department of Mathematics, Faculty of Science, King Abdul Aziz University, Jeddah, Saudi Arabia
2Department of Mathematics, Faculty of Science, King Khaled University, Abha, Saudi Arabia

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 15 May 2016; accepted 27 June 2016; published 30 June 2016
ABSTRACT
This paper proposes the combined Laplace-Adomian decomposition method (LADM) for solution two dimensional linear mixed integral equations of type Volterra-Fredholm with Hilbert kernel. Comparison of the obtained results with those obtained by the Toeplitz matrix method (TMM) demonstrates that the proposed technique is powerful and simple.
Keywords:
Singular Integral Equation, Linear Volterra-Fredholm Integral Equation, Adomian Decomposition Method, Hilbert Kernel

1. Introduction
The Volterra-Fredholm integral equation (V-FIE) arises from parabolic boundary value problems. The integral equations appear in many problems of physics and engineering. The Adomian decomposition method (ADM) was proposed by George Adomian in [1] [2] . A lot of examination work has been put as of late in applying this method to a wide range of ordinary differential equations, partial differential equations and integral equations, linear and nonlinear. Many authors discussed solutions of linear and nonlinear integral equations by utilizing different methods. What’s more, others interested singular integral equation.
We consider the linear (V-FIE) with singular kernel given by
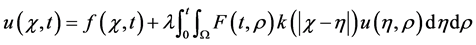 (1)
(1)
There are several techniques that have been utilized to handle the integral Equation (1) in [3] - [5] ; a few techniques, for example, the projection method, time collocation method, the trapezoidal Nystrom method, and furthermore analytical or numerical techniques were utilized to treated this equation, but this techniques experienced troubles as far as computational work utilized. In [6] treated Maleknejad and Hadizadeh Equation (1) by using the Adomian decomposition method presented in [7] [8] introduced Wazwaz [9] the modified Adomian decomposition method for solving the Volterra-Fredholm integral equations.
In this work, we display numerical techniques to obtain numerical solution for linear mixed integral equation with Hilbert kernel. In Section 2, we talk about the existence and uniqueness of the solution. In Section 3, we discuss the Adomian decomposition method, as one of the well known technique and we note that the Adomian polynomials do not appear in this work because we handle linear problems. In Section 4, we present the Laplace Adomian decomposition method and apply this method to linear mixed integral equation with Hilbert kernel. In Sections 5 and 6, we display the Toeplitz matrix method.
2. The Existence and Uniqueness of the Solution [10]
Consider the integral Equation (1), the functions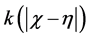 ,
, 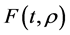 and
and 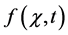 are given and called the kernel of Fredholm integral term, Volterra integral term and the free term respectively and
are given and called the kernel of Fredholm integral term, Volterra integral term and the free term respectively and 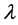 is a real parameter (may be complex and has physical meaning). Also, Ω is the domain of integration with respect to position,
is a real parameter (may be complex and has physical meaning). Also, Ω is the domain of integration with respect to position,
and the time t,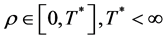 . While
. While 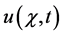 is the unknown function to be determined in the space
is the unknown function to be determined in the space
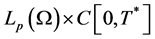 .
.
In order to guarantee the existence of a unique solution of Equation (1) we assume through this work the following conditions:
(i) The kernel of position 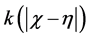 ,
,
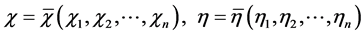
Satisfies the discontinuity condition
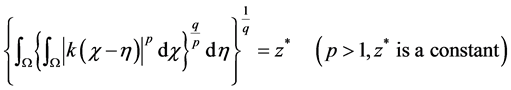
(ii) The kernel of time 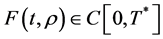 satisfies
satisfies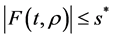 ,
, 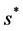 is a constant,
is a constant,
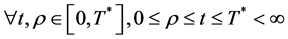 .
.
(iii) The given function  with its partial derivatives with respect to position
with its partial derivatives with respect to position  and time t is conti-
and time t is conti-
nuous in the space , and its norm is defined as
, and its norm is defined as

(iv) The unknown function  it behaves in this space, as the known function
it behaves in this space, as the known function .
.
3. The Adomian Decomposition Method for Solving Volterra-Fredholm Integral Equation [11] [12]
Adomian decomposition method [1] [2] defines the unknown function  by an infinite series
by an infinite series
 (2)
(2)
where the components  will be determined recurrently and the nonlinear term decomposed into an infinite series of Adomian polynomials
will be determined recurrently and the nonlinear term decomposed into an infinite series of Adomian polynomials

The polynomials  are produced for all kinds of nonlinearity so that
are produced for all kinds of nonlinearity so that  depends just on
depends just on ,
,  relies on upon
relies on upon  and
and , and so on. The Adomian polynomial [1] [2] ,
, and so on. The Adomian polynomial [1] [2] ,  , is given by,
, is given by,
 (3)
(3)
Substituting Equation (2) into Equation (1) to get
 (4)
(4)
The components  are computed using the following recursive relations
are computed using the following recursive relations
 (5)
(5)
 (6)
(6)
4. The Combined Laplace-Adomian Decomposition Method Applied to Volterra-Fredholm Integral Equation with Hilbert Kernel [13] - [15]
We consider the kernel  of Equation (1) take the form
of Equation (1) take the form  and applying the
and applying the
Laplace transform to both sides of Equation (1) gives:
 (7)
(7)
The linear term  will be represented by the Adomian decomposition from Equation (2). Substituting Equation (2) into Equation (7) leads to
will be represented by the Adomian decomposition from Equation (2). Substituting Equation (2) into Equation (7) leads to
 (8)
(8)
The Adomian decomposition method introduces the recursive relation

 (9)
(9)
Applying the inverse Laplace transform to the first part of Equation (9) gives . Utilizing
. Utilizing  will empower us to evaluate
will empower us to evaluate , and so on. This will prompt the complete determination of the components of
, and so on. This will prompt the complete determination of the components of  upon utilizing the second part of Equation (9). The series solution follows promptly after utilizing Equation (2). The obtained series solution may converge to an exact solution if such a solution exists.
upon utilizing the second part of Equation (9). The series solution follows promptly after utilizing Equation (2). The obtained series solution may converge to an exact solution if such a solution exists.
5. The System of Fredholm Integral Equations (SFIEs) [16] [17]
In this part, a numerical technique is used, in the integral Equation (1) to obtain a system of linear integral equa-
tions with singular kernel, so we divide the interval ,
,  as
as 
 , where
, where  Then the formula (1) reduces to SFIEs of the second kind, in the form:
Then the formula (1) reduces to SFIEs of the second kind, in the form:
 (10)
(10)
where

 (11)
(11)
where  is the error.
is the error.
6. The Toeplitz Matrix Method (TMM) [17] - [19]
In this section, we apply (TMM) to obtain the numerical solution of the SFIEs (10) with singular kernel, each equation in this system can be written in a simplify form
 (12)
(12)
The integral term in Equation (12) can be written as
 (13)
(13)
We approximate the integral in the right hand side of Equation (13) by
 (14)
(14)
where  and
and  are two arbitrary functions to be determined and
are two arbitrary functions to be determined and  is the estimate error which depends on
is the estimate error which depends on  and on the way that the coefficients
and on the way that the coefficients  are chosen. Putting
are chosen. Putting  in Equation (14) yields a set of two equations in terms of the two functions
in Equation (14) yields a set of two equations in terms of the two functions  and
and . For choosing the values of
. For choosing the values of , the error
, the error , in this case, must vanish.
, in this case, must vanish.
We can, clearly solve the result set of two equations for  and
and , to obtain
, to obtain
 (15)
(15)
 (16)
(16)
where

Hence, Equation (13) takes the form
 (17)
(17)
where

The integral Equation (12), after putting , becomes
, becomes
 (18)
(18)
The formula (18) represents a linear system of algebraic equation , where u is a vector of  elements, while
elements, while  is a matrix whose elements are given by
is a matrix whose elements are given by


The matrix  is a Toeplitz matrix of order
is a Toeplitz matrix of order , where
, where .
.
The solution of the system (18) can be obtained in the form

The error term  is determined from Equation (14) by letting
is determined from Equation (14) by letting  , to get
, to get

7. Numerical Example
Example 1: Consider the linear mixed integral equation with Hilbert kernel
 (19)
(19)
 , the exact solution
, the exact solution 
we obtain Table 1.
8. Conclusions
In this paper, we applied (LADM) for solution two dimensional linear mixed integral equations of type Volterra- Fredholm with Hilbert kernel. Additionally, comparison was made with Toeplitz matrix method (TMM). It could be concluded that (LADM) was an effective technique and simple in finding very good solutions for these sorts of equations.
Using Maple 18, we obtain Table 1 and Figure 1 (since Figure 1 represents exact solution of u at t = 0.001, λ = 0.01, N = 20).
Table 1. Results obtained for example 1 and error.


Figure 1. The exact value of u and the value of u using (LADM).
Acknowledgements
The authors would like to thank the King Abdulaziz city for science and technology.
Cite this paper
M. Salgueiro,P. González,T. F. Pena,J. C. Cabaleiro,Fatheah Ahmed Hendi,Manal Mohamed Al-Qarni, (2016) Comparison between Adomian’s Decomposition Method and Toeplitz Matrix Method for Solving Linear Mixed Integral Equation with Hilbert Kernel. American Journal of Computational Mathematics,06,177-183. doi: 10.4236/ajcm.2016.62019
References
- 1. Dean, J. and Ghemawat, S. (2008) MapReduce: Simplified Data Processing on Large Clusters. Communications of the ACM, 51, 107-133.
http://vgc.poly.edu/~juliana/courses/cs6093/Readings/dean-cacm2008.pdf - 2. Ekanayake, J., Pallickara, S. and Fox, G. (2008) MapReduce for Data Intensive Scientific Analyses. IEEE Fourth International Conference on eScience, Indianapolis, 7-12 December 2008, 277-284.
- 3. Armbrust, M., Fox, A., Griffith, R., Joseph, A.D., Katz, R.H., Konwinski, A., Lee, G., Patterson, D.A., Rabkin, A., Stoica, I. and Zaharia, M. (2009) Above the Clouds: A Berkeley View of Cloud Computing. Technical Report UCB/EECS-2009-28, EECS Department, University of California, Berkeley.
- 4. Srirama, S.N., Jakovits, P. and Vainikko, E. (2012) Adapting Scientific Computing Problems to Clouds Using MapReduce. Future Generation Computer Systems, 28, 184-192.
http://dx.doi.org/10.1016/j.future.2011.05.025 - 5. OpenStack.
http://www.openstack.org - 6. White, T. (2009) Hadoop: The Definitive Guide. O’Reilly Media.
- 7. DevStack.
http://devstack.org - 8. Amazon Web Services: Elastic MapReduce.
http://aws.amazon.com/elasticmapreduce - 9. Riteau, P., Iordache, A. and Morin, C. (2011) Resilin: Elastic MapReduce for private and community Clouds. Research Report RR-7767, INRIA.
- 10. OpenStack: Project Sahara.
https://wiki.openstack.org/wiki/Sahara - 11. Loughran, S., Alcaraz Calero, J.M., Farrell, A., Kirschnick, J. and Guijarro, J. (2012) Dynamic Cloud Deployment of a MapReduce Architecture. IEEE Internet Computing, 16, 40-50.
http://dx.doi.org/10.1109/MIC.2011.163 - 12. Liu, H. and Orban, D. (2011) Cloud MapReduce: A MapReduce Implementation on Top of a Cloud Operating system. 11th IEEE/ACM International Symposium on Cluster, Cloud and Grid Computing, Washington DC, 23-26 May 2011, 464-474.
- 13. Apache Cloudstack.
http://cloudstack.apache.org - 14. Moreno-Vozmediano, R., Montero, R.S. and Llorente, I.M. (2012) IaaS Cloud Architecture: From Virtualized Datacenters to Federated Cloud Infrastructures. IEEE Computer, 45, 65-72.
http://dx.doi.org/10.1109/MC.2012.76 - 15. Nurmi, D., Wolski, R., Grzegorczyk, C., Obertelli, G., Youseff, L. and Zagorodnov, D. (2009) The Eucalyptus Open-Source Cloud-Computing System. 9th IEEE International Symposium on Cluster Computing and the Grid, Shanghai, 18-21 May 2009, 124-131.
- 16. GridGain Systems. GridGain 3.0—High Performance Cloud Computing Whitepaper. Technical Report, 2011.
- 17. Ekanayake, J., Li, H., Zhang, B., Gunarathne, T., Bae, S.-H., Qiu, J. and Fox, G. (2010) Twister: A Runtime for Iterative MapReduce. Proceedings of the 19th ACM International Symposium on High Performance Distributed Computing, Chicago, 21-25 June 2010, 810-818.
- 18. Apache Hadoop 1.0.4 Based on CentOS 6.3 VM.
https://drive.google.com/file/d/0B2lmVzXW-C5UcmZIYk80dTZJb0k/edit?usp=sharing - 19. Qosh Main Page.
https://code.google.com/p/quick-openstacked-hadoop - 20. Adomian, G. (1994) Solving Frontier Problems of Physics: The Decomposition Method. Springer, Netherlands.
http://dx.doi.org/10.1007/978-94-015-8289-6 - 21. Adomian, G. (1988) A Review of the Decomposition Method in Applied Mathematics. Journal of Mathematical Analysis and Applications, 135, 501-544.
http://dx.doi.org/10.1016/0022-247X(88)90170-9 - 22. Hacia, L. (1996) On Approximate Solution for Integral Equations of Mixed Type. ZAMM—Journal of Applied Mathematics and Mechanics, 76, 415-416.
- 23. Kauthen, P.J. (1989) Continuous Time Collocation Methods for Volterra-Fredholm Integral Equations. Numerische Mathematik, 56, 409-424.
http://dx.doi.org/10.1007/BF01396646 - 24. Han, G.Q. and Zhang, L.Q. (1994) Asymptotic Expansion for the Trapezoidal Nystrom Method of Linear Volterra-Fredholm Equations. Journal of Computational and Applied Mathematics, 51, 339-348.
http://dx.doi.org/10.1016/0377-0427(92)00013-Y - 25. Maleknejad, K. and Hadizadeh, M. (1999) A New Computational Method for Volterra-Fredholm Integral Equations. Journal of Computational and Applied Mathematics, 37, 1-8.
http://dx.doi.org/10.1016/S0898-1221(99)00107-8 - 26. Adomian, G. (1991) A Review of the Decomposition Method and Some Recent Results for Nonlinear Equation. Computers & Mathematics with Applications, 21, 101-127.
http://dx.doi.org/10.1016/0898-1221(91)90220-X - 27. Cherruault, Y., Saccomandi, G. and Some, B. (1992) New Results for Convergence of Adomian’s Method Applied to Integral Equations. Mathematical and Computer Modelling, 16, 85-93.
http://dx.doi.org/10.1016/0895-7177(92)90009-A - 28. Wazwaz, A.M. (1999) A Reliable Modification of Adomian’s Decomposition Method. Applied Mathematics and Computation, 102, 77-86.
http://dx.doi.org/10.1016/S0096-3003(98)10024-3 - 29. Abdou, M.A., Hendi, F.A. and Abu Alnaja, K.M. (2012) Computational Method for Solving Mixed Linear Integral Equation. International Journal of Numerical Methods and Applications, 7, 85-106.
- 30. Jafari, H., Tayyebi, E., Sadeghi, S. and Khalique, C.M. (2014) A New Modification of the Adomian Decomposition Method for Nonlinear Integral Equations. International Journal of Advances in Applied Mathematics and Mechanics, 1, 33-39.
- 31. Hendi, F.A. and Bakodah, H.O. (2012) Numerical Solution of Fredholm-Volterra Integral Equation in Two Dimensonal Space By Using Discrete Adomian Decomposition Method”, IJRRAS, 10(3), pp. 466-471,.
- 32. Wazwaz, A.M. (2012) The Combined Laplace Transform-Adomian Decomposition Method for Handling Nonlinear Volterra Integro-Differential Equations. Applied Mathematics and Computation, 216, 1304-1309.
http://dx.doi.org/10.1016/j.amc.2010.02.023 - 33. Wazwaz, A.M. and Mehanna, M.S. (2010) The Combined Laplace-Adomian Method for Handling Singular Integral Equation of Heat Transfer. International Journal of Nonlinear Sciences, 10, 248-252.
- 34. Hendi, F.A. (2011) Laplace Adomian Decomposition Method for Solving the Nonlinear Volterra Integral Equation with Weakly Kernels. Studies in Nonlinear Sciences, 2, 129-134.
- 35. Abdou, M.A., El-Kalla, I.L. and Al-Bugami, A.M. (2011) Numerical Solution for Volterra-Ferdholm Integral Equation with a Generalized Singular Kernel. Journal of Modern Methods in Numerical Mathematics, 2, 1-15.
http://dx.doi.org/10.20454/jmmnm.2011.60 - 36. Al-Bugami, A.M. (2013) Toeplitz Matrix Method and Volterra-Hammerstien Integral Equation with a Generalized Singular Kernel. Progress in Applied Mathematics, 6, 16-42.
- 37. Abdou, M.A. and Hendi, F.A. (2005) Numerical Solution of Fredholm Integral Equation with Hilbert Kernel. The KSIAM Journal, 9, 111-123.
- 38. Abdou, M.A., Mohamed, K.I. and Ismail, A.S. (2003) On the Numerical Solution of Fredholm-Voltrra Integral Equation. Journal of Applied Mathematics and Computing, 146, 713-728.
http://dx.doi.org/10.1016/S0096-3003(02)00615-X
上一篇:On the Location of Zeros of Po 下一篇:High Energy Physics and Cosmol
最新文章NEWS
- Auto-Bäcklund Transformation and Extended Tanh-Function Methods to Solve the Time-Dependent Coeffici
- A Third-Order Scheme for Numerical Fluxes to Guarantee Non-Negative Coefficients for Advection-Diffu
- Conjugate Effects of Radiation and Joule Heating on Magnetohydrodynamic Free Convection Flow along a
- An O(k<sup>2</sup>+kh<sup>2</sup>+h<sup>2</sup>) Accurate Two-le
- On the Location of Zeros of Polynomials
- Peristaltic Pumping of a Conducting Sisko Fluid through Porous Medium with Heat and Mass Transfer
- An Accurate Numerical Integrator for the Solution of Black Scholes Financial Model Equation
- Simulation of Time-Dependent Schrödinger Equation in the Position and Momentum Domains
推荐期刊Tui Jian
- Chinese Journal of Integrative Medicine
- Journal of Genetics and Genomics
- Journal of Bionic Engineering
- Pedosphere
- Chinese Journal of Structural Chemistry
- Nuclear Science and Techniques
- 《传媒》
- 《哈尔滨师范大学自然科学学报》
热点文章HOT
- Asymptotic Solutions for the Fifth Order Critically Damped Nonlinear Systems in the Case for Small E
- Partial Fraction Decomposition by Repeated Synthetic Division
- Higher-Order Numerical Solution of Two-Dimensional Coupled Burgers’ Equations
- Group Method Analysis of MHD Mixed Convective Flow Past on a Moving Curved Surface with Suction
- Simple and Multi Linear Regression Model of Verbs in Quran
- Peristaltic Pumping of a Conducting Sisko Fluid through Porous Medium with Heat and Mass Transfer
- Conjugate Effects of Radiation and Joule Heating on Magnetohydrodynamic Free Convection Flow along a
- An O(k<sup>2</sup>+kh<sup>2</sup>+h<sup>2</sup>) Accurate Two-le

