Global Analysis of Solutions of a New Class of Rational Difference Equation
Vol.07No.04(2017), Article ID:81156,9 pages
10.4236/ajcm.2017.74036
O. Moaaz1, E. M. Elabbasy1, Sh. Alsaeed2
1Department of Mathematics, Faculty of Science, Mansoura University, Mansoura, Egypt
2Department of Mathematics, The Faculty of Education, Al al-Bayt University, Mafraq, Jordan
Copyright © 2017 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: July 28, 2017; Accepted: December 16, 2017; Published: December 19, 2017
ABSTRACT
The study suggests asymptotic behavior of the solution to a new class of difference equations: . where and are positive real numbers for , and the initial conditions are randomly positive real numbers where . Accordingly, we consider the stability, boundedness and periodicity of the solutions of this recursive sequence. Indeed, we give some interesting counter examples in order to verify our strong results.
Keywords:
Difference Equation, Stability, Boundedness, Globale Stability and Periodicity

1. Introduction
The global asymptotic behavior of the solutions and oscillation of solution are two such qualitative properties which are very important for applications in many areas such as control theory, mathematical biology, neural networks, etc. It is impossible to use computer based (numerical) techniques to study the oscillation or the asymptotic behavior of all solutions of a given equation due to the global nature of these properties. Therefore, these properties have received the attention of several mathematicians and engineers.
Currently, much attention has given to study the properties of the solutions of the recursive sequences from scientists in various disciplines. Specifically, the topics dealt with include the following:
- Finding equilibrium points for the recursive sequences;
- Investigating the local stability of the solutions of the recursive sequences;
- Finding conditions which insure that the solutions of the recursive sequences are bounded;
- Investigating the global asymptotic stability of the solutions of the recursive sequences;
- Finding conditions which insure that the solutions of equation are periodic with positive prime period two or more;
- Finding conditions for oscillation of solutions.
Closely related global convergence results were well-gained from these articles [1] - [25] . Khuong in [14] studied the dynamics the recursive sequences
For further related and special cases of this difference equations see [4] [5] [6] , [21] [22] [24] .
Elsayed [9] studied the periodicity, the boundedness of the positive solution of the recursive sequences
Abdelrahman [1] considered analytical investigation of the solution of the recursive sequence
By new method, Elsayed [10] investigated the periodic solution of the equation
Also, Moaaz [18] completed the results of [10] .
In this work, we deal with some qualitative behaviour of the solutions of the recursive sequence
(1.1)
where and are positive real numbers for , and the the initial conditions are arbitrary positive real numbers where .
In the next, we will and to many of the basic concepts. Before anything, the concept of equilibrium point is essential in the study of the dynamics of any physical system. A point in the domain of the function is called an equilibrium point of the equation
(1.2)
if is a fixed point of [ ]. For a stability of equilibrium point, equilibrium point of equation (2) is said to be locally stable if for all there exists such that, if for with . As well, is said to be locally asymptotically stable if it is locally stable and there exists such that, if for with , then . Also, is said to be a global attractor if used for every for , we have . On the other hand, is said to be unstable if it is not locally stable.
Finally, Equation (1.2) is called permanent and bounded if there exists numbers r and R with such that for any initial conditions for there exists a positive integer N which depends on these initial conditions such as for all .
The linearized equation of Equation (1.1) about the equilibrium point is
(1.3)
where
Theorem 1.1. [15] Assume that for . The equilibrium of (1.1) is locally asymptotically stable if
(1.4)
2. Local Stability of Equation (1.1)
The equilibrium point of Equation (1.1) is
and so,
where
Let defined by
(2.1)
Therefore it follows that
(2.2)
and
(2.3)
for .
Theorem 2.1. Let be equilibrium of Equation (1.1). If
than is locally stable.
Proof. From (2.2) to (2.3), we obtain
and
for . Thus, the linearized equation of (1.1) is
It follows by Theorem 1.1 that Equation (1.1) is locally stable if
where , and hence,
Thus, we find
and so,
Hence, the proof is complete.
□
In order to verify and support our theoretical outcomes and discussions, in this concern, we investigate several interesting numerical examples.
Example 2.1. By Theorem 2.1, the equilibrium Equation (1.1) with , , , and , is locally stable (see Figure 1).
3. Global Stability of Equation (1.1)
In the following theorem, we check into the global stability of the recursive sequence (1.1).
Theorem 3.1. The equilibrium of Equation (1.1) is global attractor if

Figure 1. The stable solution corresponding to difference Equation (1.1).
Proof. We consider the function as follow:
From (2.2) and (2.3), we note that is increasing in and decreasing in for all . Suppose that is a solution of the system
Then, we find
and
Hence, we get
(3.1)
and
(3.2)
By (3.1) and (3.2), we obtain
Thus,
Since , we have that . Hence, the proof of Theorem 3.1 is complete. □
4. Periodic Solutions
In this section, we enumerate some basic facts concerning the existence of two period solutions.
Theorem 4.1. Equation (1.1) has prime period-two solutions if
(4.1)
Proof. Assume that Equation (1.1) has a prime period-two solution
We shall prove that condition (4.1) holds. From Equation (1.1), we see that
and hence,
Thus, we get
(4.2)
and
(4.3)
From (4.3) and (4.2), we have
Dividing , then we find
(4.4)
By combining (4.2) and (4.3), we obtain
Since , we get
(4.5)
Now, evident is that (4.4) and (4.5) that and are both two positive distinct roots of the quadratic equation
(4.6)
Hence, we obtain
which has the same extent as
Hence, the proof is complete. □
The next numerical example is mimicry to enhance our results.
Example 4.1. By Theorem 4.1, Equation (1.1) with , , , , and , has prime period two solution (see Figure 2)
5. Boundedness
Theorem 5.1. Every solution of Equation (1.1) is bounded and persists.
Proof. Let be a Solution (1.1), we can conclude from (1.1) that
Then
Also, from Equation (1.1), we see that
then,
Thus, the solution is bounded and persists and the proof is complete. □
Conclusion 1. In this paper, we study a asymptotic behavior of solutions of a general class of difference Equation (1.1). Our results extend and generalize to the earlier ones. Moreover, we obtain the next results:
- The equilibrium point of Equation (1.1) is local stable if . Also, if , then is global attractor.
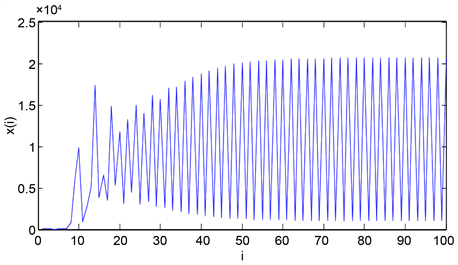
Figure 2. Prime period two solution of Equation (1.1).
- Equation (1.1) has a prime period-two solutions if .
- Every solution of (1.1) is bounded and persists.
Acknowledgements
The author is very grateful to the reviewers for their valuable suggestions and useful comments on this paper.
Cite this paper
Moaaz, O., Elabbasy, E.M. and Alsaeed, S. (2017) Global Analysis of Solutions of a New Class of Rational Difference Equation. American Journal of Computational Mathematics, 7, 495-503. https://doi.org/10.4236/ajcm.2017.74036
References
- 1. Abdelrahman, M.A.E. and Moaaz, O. (2017) Investigation of the New Class of the Nonlinear Rational Difference Equations. Fundamental Research and Development International, 7, 59-72.
- 2. Abdelrahman, M.A.E. and Moaaz, O. (2018) On the New Class of the Nonlinear Difference Equations. Electronic Journal of Mathematical Analysis and Applications, 6, 117-125.
- 3. Agarwal, R. (1992) Difference Equations and Inequalities. Tteory, Methods and Applications, Marcel Dekker Inc., New York.
- 4. Amleh, A.M., Grove, E.A., Georgiou, A. and Ladas, G. (1999) On the Recursive Sequence ωη+1 = α + ωη-1/ωη. The Journal of Mathematical Analysis and Applications, 233, 790-798. https://doi.org/10.1006/jmaa.1999.6346
- 5. Berenhaut, K.S. and Stevic, S. (2006) The Behaviour of the Positive Solutions of the Difference Equation ψη = a + (ψη-2/ψη-1)σ. Journal of Difference Equations and Applications, 12, 909-918. https://doi.org/10.1080/10236190600836377
- 6. Camouzis, E., DeVault, R. and Ladas, G. (2001) On the Recursive Sequence ωη+1 = -1 + ωη-1/ωη. Journal of Difference Equations and Applications, 7, 477-482.https://doi.org/10.1080/10236190108808283
- 7. Elabbasy, E.M., EL-Metwally, H.A. and Elsayed, E.M. (2006) On the Difference Equation ωη+1 = aωη - bωη/(cωη - dωη-1). Advances in Difference Equations, 2006, 1-10. https://doi.org/10.1155/ADE/2006/82579
- 8. Elaydi, S.N. (1996) An Introduction to Difference Equations, Undergraduate Texts in Mathematics. Springer, New York. https://doi.org/10.1007/978-1-4757-9168-6
- 9. Elsayed, E.M. and El-Metwally, H.A. (2012) Qualitative Studies of Scalars and Systems of Difference Equations. Publisher LAP Lambert Academic, Saarbrücken.
- 10. Elsayed, E.M. (2015) New Method to Obtain Periodic Solutions of Period Two and Three of a Rational Difference Equation. Nonlinear Dynamics, 79, 241-250.https://doi.org/10.1007/s11071-014-1660-2
- 11. Elsayed, E.M. (2016) Dynamics and Behavior of a Higher Order Rational Difference Equation. The Journal of Nonlinear Sciences and Applications, 9, 1463-1474.
- 12. Elsayed, E.M. (2010) On the Global Attractivity and the Periodic Character of a Recursive sequence. Opuscula Mathematica, 30, 431-446.https://doi.org/10.7494/OpMath.2010.30.4.431
- 13. Grove, E.A. and Ladas, G. (2005) Periodicities in Nonlinear Difference Equations, Vol. 4. Chapman & Hall/CRC, London.
- 14. Khuong, V.V. (2008) On the Positive Nonoscillatory Solution of the Difference Equations ωη+1 = α + (ωη-k/ωη-r)p. Applied Mathematics-A Journal of Chinese Universities, 24, 45-48.
- 15. Kulenovic, M.R.S. and Ladas, G. (2001) Dynamics of Second Order Rational Difference Equations with Open Problems and Conjectures. Chapman & Hall/CRC, Florida. https://doi.org/10.1201/9781420035384
- 16. El-Metwally, H., Ladas, G., Grove, E.A. and Voulov, H.D. (2001) On the Global Attractivity and the Periodic Character of Some Difference Equations. Journal of Difference Equations and Applications, 7, 837-850. https://doi.org/10.1080/10236190108808306
- 17. Metwally, H.E., Grove, E.A. and Ladas, G. (2000) A Global Convergence Result with Applications to Periodic Solutions. Journal of Mathematical Analysis and Applications, 245, 161-170. https://doi.org/10.1006/jmaa.2000.6747
- 18. Moaaz, O. (2016) Comment on New Method to Obtain Periodic Solutions of Period Two and Three of a Rational Difference Equation. Nonlinear Dynamics, 79, 241-250. https://doi.org/10.1007/s11071-016-3293-0
- 19. Moaaz, O. and Abdelrahman, M.A.E. (2016) Behaviour of the New Class of the Rational Difference Equations. Journal of Mathematical Analysis and Applications, 4, 129-138.
- 20. Moaaz, O. and Abdelrahman, M.A.E. (2017) On the Class of the Rational Difference Equations. International Journal of Advances in Mathematics, 2017, 46-55.
- 21. Ocalan, O. (2014) Dynamics of the Difference Equation ωη+1 = Pη + ωη-k/ωη with a Period-Two Coefficient. Applied Mathematics and Computation, 228, 31-37. https://doi.org/10.1016/j.amc.2013.11.020
- 22. Saleh, M. and Aloqeili, M. (2005) On the Difference Equation ψη+1 = A + ψη/ψη-k. Applied Mathematics and Computation, 171, 862-869. https://doi.org/10.1016/j.amc.2005.01.094
- 23. Stevic, S. (2005) On the Recursive Sequence ψη+1 = a + βψη-k/f(ψη,···, ψη-k+1). Taiwanese Journal of Mathematics, 9, 583-593.
- 24. Sun, T. and Xi, H. (2007) On Convergence of the Solutions of the Difference Equation ωη+1 = 1 + ψη/ψη-k. Journal of Mathematical Analysis and Applications, 325, 1491-1494. https://doi.org/10.1016/j.jmaa.2006.02.080
- 25. Zhang, L., Zhang, G. and Liu, H. (2005) Periodicity and Attractivity for a Rational Recursive Sequence. Journal of Applied Mathematics and Computing, 19, 191-201. https://doi.org/10.1007/BF02935798
上一篇:On the Location of Zeros of Po 下一篇:Comparison of Numerical Approx
最新文章NEWS
- Auto-Bäcklund Transformation and Extended Tanh-Function Methods to Solve the Time-Dependent Coeffici
- A Third-Order Scheme for Numerical Fluxes to Guarantee Non-Negative Coefficients for Advection-Diffu
- Conjugate Effects of Radiation and Joule Heating on Magnetohydrodynamic Free Convection Flow along a
- An O(k<sup>2</sup>+kh<sup>2</sup>+h<sup>2</sup>) Accurate Two-le
- On the Location of Zeros of Polynomials
- Peristaltic Pumping of a Conducting Sisko Fluid through Porous Medium with Heat and Mass Transfer
- An Accurate Numerical Integrator for the Solution of Black Scholes Financial Model Equation
- Simulation of Time-Dependent Schrödinger Equation in the Position and Momentum Domains
推荐期刊Tui Jian
- Chinese Journal of Integrative Medicine
- Journal of Genetics and Genomics
- Journal of Bionic Engineering
- Pedosphere
- Chinese Journal of Structural Chemistry
- Nuclear Science and Techniques
- 《传媒》
- 《哈尔滨师范大学自然科学学报》
热点文章HOT
- Asymptotic Solutions for the Fifth Order Critically Damped Nonlinear Systems in the Case for Small E
- Partial Fraction Decomposition by Repeated Synthetic Division
- Higher-Order Numerical Solution of Two-Dimensional Coupled Burgers’ Equations
- Group Method Analysis of MHD Mixed Convective Flow Past on a Moving Curved Surface with Suction
- Simple and Multi Linear Regression Model of Verbs in Quran
- Peristaltic Pumping of a Conducting Sisko Fluid through Porous Medium with Heat and Mass Transfer
- Conjugate Effects of Radiation and Joule Heating on Magnetohydrodynamic Free Convection Flow along a
- An O(k<sup>2</sup>+kh<sup>2</sup>+h<sup>2</sup>) Accurate Two-le
