Modified Ramsey Rule and Optimal Carbon Tax
Vol.06No.02(2016), Article ID:65572,6 pages
10.4236/acs.2016.62022
Masayuki Otaki
Institute of Social Sciences, The University of Tokyo, Tokyo, Japan

Copyright © 2016 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 12 March 2016; accepted 15 April 2016; published 18 April 2016
ABSTRACT
The Ramsey rule is regarded as a convenient vehicle for estimating the social discount rate in general. Carbon pricing is treated as another theory of environmental economics. This study clarifies the theoretical relationship between the Ramsey rule and optimal carbon price, which has been overlooked in the existing research. It succeeds in deriving the optimal carbon price from the modified Ramsey rule in stationary state. Since the Ramsey rule decides the dynamics of an economy and a stationary state is its destination, by using the optimization condition of individual who are assumed to live infinitesimally short life, we can solve the optimal carbon price at stationary state.
Keywords:
Modified Ramsey Rule, Optimal Carbon Price, Social Discount Rate, Carbon Cycle

1. Introduction
Many studies utilize the Ramsey rule for estimating appropriate social discount rate (e.g., Cline [1] ; Stern [2] ; Nordhaus [3] ). However, as Kuninori and Otaki [4] point out, the causality presumed in existing studies is incorrect. A natural interpretation of optimal growth theory leads us to the following proper causality of the Ramsey rule. That is, consumption gradually increases when the rate of interest exceeds the rate of time preference because an individual is rewarded by higher utility from postponing consumption.
Since the Ramsey rule shows optimal consumption and/or investment path, if an original model is devised to include a negative intertemporal externality, it is evident that optimal carbon price can be calculated based on this formula. This study analyzes the relationship between the Ramsey rule and optimal carbon price and calculates such a price in reality.
It should be noted that the Ramsey rule evaluated in stationary state implies the tangency condition of optimality, that is, equalization of the marginal rate of substitution in consumption or production with the relative price of carbon. Unless such a condition is upheld in the long run, there is a room for improving utility. Accordingly, as far as the stationary state is optimal, the foregoing tangency condition can be induced from the Ramsey rule; thus, an integrated view is obtained on how the traditional estimation of the social discount rate based on the Ramsey rule is related to the optimal carbon pricing.
The rest of this paper is organized as follows. Section 2 reveals the relationship between the modified Ramsey rule and the optimal carbon price using Kuninori and Otaki [4] and Otaki [5] . One will find that the characteristic of the carbon cycle equation plays a crucial role in determining the carbon price. Section 3 provides brief concluding remarks.
2. Modified Ramsey Rule and Carbon Price
Based on Kuninori and Otaki [4] and Otaki [5] , this section derives the optimal carbon price from the modified Ramsey rule.
2.1. Intertemporal Negative Externality in Utility Function
Throughout this study, it is assumed that there is a negative intertemporal externality in the world economy originating from excess emissions of carbon dioxide (CO2). In this subsection let us consider the case in which such a negative externality directly affects people’s utility. The concave instantaneous utility function, u, is assumed to be
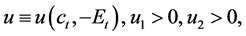 (1)
(1)
ct and Et denote consumption and accumulated emissions (measured by CO2 tonnage), respectively. ui is the i-th partial derivative of the utility function.
The dynamics of the carbon cycle are assumed to be
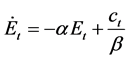 , (2)
, (2)
where  denotes the absorption ratio of CO2 by the Earth.
denotes the absorption ratio of CO2 by the Earth.  denotes the efficiency of production measured by the amount of production (consumption) that can be produced by unit emissions1.
denotes the efficiency of production measured by the amount of production (consumption) that can be produced by unit emissions1.
The optimization problem is represented as follows:
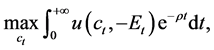 subject to (2). (3)
subject to (2). (3)
The corresponding Hamiltonian, 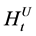 , is
, is
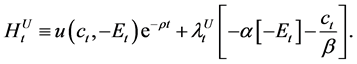 (4)
(4)
The necessary and sufficient conditions for the maximization are Equation (2) and
 (5)
(5)
Differentiating the top equation in (5) logarithmically, yields
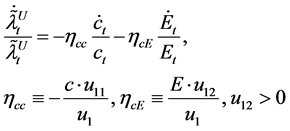 (6)
(6)
Substituting Equation (6) into the middle equation in (5), it can be ascertained that the following relation holds:
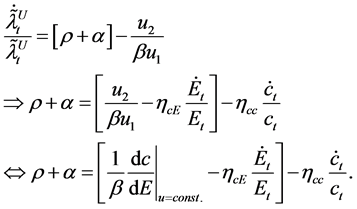 (7)
(7)
The bottom equation in (7) is the modified Ramsey rule when a negative externality exists in the utility function. The economic implication is as follows: the left-hand side of the equation represents the effective social discount rate. The effectiveness implies that the absorption ratio,  , is added to the discount rate. This is because emitted CO2 becomes harmless with the ratio,
, is added to the discount rate. This is because emitted CO2 becomes harmless with the ratio,  , at every moment and a social planner can be permitted to discount future damages originating from global warming at a higher rate than the rate of time preference, ρ.
, at every moment and a social planner can be permitted to discount future damages originating from global warming at a higher rate than the rate of time preference, ρ.
The first bracket of the right-hand side of the modified Ramsey rule (the bottom equation in (7)) corresponds
to the net marginal benefit in terms of utility. 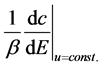 denotes the marginal substitution rate of consump-
denotes the marginal substitution rate of consump-
tion to emissions, which represents the utility obtained from unit emissions. When β is large, people can enjoy high consumption with less emissions. Accordingly, ceteris paribus, the obtained marginal utility decreases due
to the saturation of consumption. 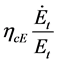 is the marginal disutility from emissions; summing them, the net mar-
is the marginal disutility from emissions; summing them, the net mar-
ginal utility originating from emissions is obtained.
The second term of the right-hand side of the modified Ramsey rule (the bottom equation in (7)) indicates the optimal path of consumption. The optimal consumption decreases proportionately to the difference between the effective social discount rate and the net marginal utility obtained by emissions. In other words, as people become more impatient or marginal utility from emissions more attractive, the optimal consumption stream is concentrated in the near future; and thus, decreases as time goes by. The dynamics of the economy are fully described by the differential Equations (2) and (7).
Thus, we have established the modified Ramsey rule wherein a negative intertemporal externality originating from CO2 emissions directly affects people’s utility function. Using Equation (7), let the optimal carbon price be calculated in the stationary state as follows:
By the definition of stationary state,  holds, which yields
holds, which yields
 . (8)
. (8)
Because the left-hand side of Equation (8) is the marginal substitution rate of consumption to emissions reduction (i.e., the tangency of an indifference curve in the  plane), the right-hand side of Equation (8) in-
plane), the right-hand side of Equation (8) in-
dicates the inverse of the optimal carbon price in terms of unit emissions. That is, people should pay 
times of money for consumption in exchange for the social cost incurred by unit emissions. This result coincides with that of Otaki [5] .
2.2. Intertemporal Negative Externality in Production Function
In this subsection, we derive the modified Ramsey rule wherein a negative intertemporal externality affects the production function. Let the strictly concave production function, F, be denoted as
 , (9)
, (9)
where yt is total output of goods, and kt denotes input of goods for production. It is assumed that anthropogenic combustion of fossil fuels concentrates CO2 emissions and lowers productivity. Accordingly, the partial derivative of the production function is positive. In such a case, the differential equation that describes the carbon cycle becomes
 . (10)
. (10)
The optimization problem to be solved is
 , subject to Equations (8) and (9), (11)
, subject to Equations (8) and (9), (11)
where v is a strictly concave instantaneous utility function. The corresponding Hamiltonian,  , is
, is
 . (12)
. (12)
The necessary-sufficient conditions are Equation (10) and
 (13)
(13)
Combining the middle equation in (13) with the top equation, yields
 (14)
(14)
Combining the two equations in (14), we obtain
 (15)
(15)
where  is defined as
is defined as
 . (16)
. (16)
Equation (15) corresponds to the modified Ramsey rule. The left-hand side of this equation represents the effective social discount rate as in Equation (8). The first term on the right-hand side of Equation (15) is the marginal substitution rate of emissions to intermediate goods, which means the marginal benefit of emissions in conjunction with economizing intermediate goods. The second term on the right-hand side of Equation (15) denotes the increase rate of marginal propensity to consume. If this rate is heightened, this saves more artificial production resources; and thereby improves the efficiency of production. The third term on the right-hand side of Equation (14) implies the decrease in marginal utility in proportion to the increase rate of consumption. Future consump-
tion increases monotonously if the marginal benefit of emissions,  , exceeds the rate of tolerance
, exceeds the rate of tolerance
to current consumption, . Hence, it can be ascertained that Equation (15) is the modified Ramsey rule wherein an intertemporal negative externality exists within the production process.
. Hence, it can be ascertained that Equation (15) is the modified Ramsey rule wherein an intertemporal negative externality exists within the production process.
The carbon price relative to the unit emission reducing cost at the stationary state can be solved by letting
 . This yields
. This yields
 . (17)
. (17)
The profit-maximization condition implies
 , (18)
, (18)
where p is the carbon price (i.e. goods price) in terms of unit emissions reducing cost. Accordingly, by combining Equations (17) and (18), the optimal carbon price, p, is solved as
 . (19)
. (19)
It is evident from the discussion in Subsection 2.1, that the optimal carbon price is invariant with where the negative externality exists.
2.3. Kuninori-Otaki’s [4] Case
Kuninori and Otaki [4] is the first study that finds some modification is necessary for the Ramsey rule to be tenable for analyzing the global warming problem. Their model differs from the foregoing two models in this paper on the point that it includes a capital accumulation process and that a negative externality emerges from capital accumulation. This is because the authors assume that the overall production capacity is determined by capital stock and excess emissions stem from the production process. Although the model is transformed in order to include capital accumulation, one can ascertain the similar formula concerning the optimal carbon price derived as follows.
Kuninori and Otaki [4] assume the following negative externality in the concave production function:
 (20)
(20)
The maximization problem is
 , subject to
, subject to . (21)
. (21)
The corresponding Hamiltonian HK is defined as
 . (22)
. (22)
The necessary-sufficient conditions for optimality are
 (23)
(23)
Combining the top and middle equations in (23), the following modified Ramsey rule is obtained. That is,
 (24)
(24)
where Ψ* is the optimal carbon tax rate, and π denotes the average productivity of capital. This corresponds to the modified Ramsey rule in Kuninori and Otaki [4] .
From the static profit maximization condition, as far as one wishes to achieve the first-best allocation by a market economy,
 (25)
(25)
holds. The optimal carbon price of a consumption goods measured by unit social emissions cost incurred by capital accumulation in the stationary state satisfies
 , (26)
, (26)
where p* is the optimal relative carbon price (or goods price). Comparing Equation (26) with Equation (19), it is found that Kuninori and Otaki [4] correspond to the case in which ,
, . This is evident from the structure of the model that Kuninori and Otaki [4] regards the absorption rate by the earth,
. This is evident from the structure of the model that Kuninori and Otaki [4] regards the absorption rate by the earth,  , as zero and set the parameter of the carbon efficiency, β, to unity. Accordingly, Equation (26) is a special case of Equation (19); thus, in this case too, we succeed in driving the optimal carbon price from the corresponding modified Ramsey rule.
, as zero and set the parameter of the carbon efficiency, β, to unity. Accordingly, Equation (26) is a special case of Equation (19); thus, in this case too, we succeed in driving the optimal carbon price from the corresponding modified Ramsey rule.
To summarize our assertion, since each modified Ramsey rule represents the optimal consumption/emissions path, it is clear that it is possible to calculate the optimal carbon price in the stationary state which is the destination of the optimal path.
3. Concluding Remarks
This study analyzed the relationship between the modified Ramsey rule and the optimal carbon price levied on consumption goods. Regardless of the origin of an intertemporal negative externality, the modified Ramsey rule solves the optimal carbon price in the stationary state, p, as
 . (27)
. (27)
When the rate of time preference, ρ, is high and people are impatient, the carbon price becomes lower because such an economy deeply discounts future damages. If the earth’s absorption capacity of emission is higher and takes a larger value, the carbon price is lowered. This is because emitted CO2 does not stay within the atmosphere for a long time and is less harmful. Finally, the larger emissions efficiency, which corresponds to a high value of β, the less emissions are necessary for producing goods. Accordingly, it is acceptable to reduce the carbon price in such a case.
takes a larger value, the carbon price is lowered. This is because emitted CO2 does not stay within the atmosphere for a long time and is less harmful. Finally, the larger emissions efficiency, which corresponds to a high value of β, the less emissions are necessary for producing goods. Accordingly, it is acceptable to reduce the carbon price in such a case.
Acknowledgements
This paper owes much to the discussions with Professor Morio Kuninori. I am thankful for the warm-heartedness of him and staffs of DBJ Research Center on Global Warming. In addition, the research is funded by MEXT/ JSPS KAKENHI Grant Number 60183761.
Cite this paper
Masayuki Otaki, (2016) Modified Ramsey Rule and Optimal Carbon Tax. Atmospheric and Climate Sciences,06,267-272. doi: 10.4236/acs.2016.62022
References
- 1. Cline, W.R. (1992) The Economics of Global Warming. Institute for International Economics, Washington DC.
- 2. Stern, N. (2007) The Economics of Climate Change: The Stern Review. Cambridge University Press, Cambridge, UK.
http://dx.doi.org/10.1017/CBO9780511817434 - 3. Nordhaus, W. (2013) The Climate Casino: Risk, Uncertainty, and Economics for a Warming World. Yale University Press, New Haven, CT.
- 4. Kuninori, M. and Otaki, M. (2016) Modified Ramsey Rule, Optimal Carbon Tax, and Economic Growth. Atmospheric and Climate Sciences, 6, 224-235.
http://dx.doi.org/10.4236/acs.2016.62019 - 5. Otaki, M. (2016) Properties of the Social Discount Rate and Intertemporal Negative Externality in the Utility or Production Function. Low Carbon Economy, 7, 47-53.
http://dx.doi.org/10.4236/lce.2016.71005
NOTES

1Another type of equation of the carbon cycle is examined in Subsection 2.3.
上一篇:Assessment of Regional Climate 下一篇:Regional Profiles and Precipit
最新文章NEWS
- Assessment of Regional Climate Models over Côte D'Ivoire and Analysis of Future Projections ov
- Physicochemical Characteristics of Water Samples Collected from River Sokoto, Northwestern Nigeria
- Correlation between Atmospheric Temperature and Soil Temperature: A Case Study for Dhaka, Bangladesh
- Convective Rainfall in Amazonia and Adjacent Tropics
- Understanding and Forecasting Zonda Wind (Andean Foehn) in Argentina: A Review
- Study Pressure Fields Affecting Cyclone Rainfall: Case Study of Iran
- Fair Plan 6: Quo Vadis the 80%-Emission-Reduction-By-2050 Plan?
- Assessment of Selective Reduction Strategies over Northeastern Regions of Ohio Using a Photochemical
推荐期刊Tui Jian
- Chinese Journal of Integrative Medicine
- Journal of Genetics and Genomics
- Journal of Bionic Engineering
- Pedosphere
- Chinese Journal of Structural Chemistry
- Nuclear Science and Techniques
- 《传媒》
- 《哈尔滨师范大学自然科学学报》
热点文章HOT
- The Introduction of Specific Water and Maximum Airborne Specific Water & the Improvement of Dynamic
- Emission of Combustible Gases at Traffic and Practising Waste Dumpsite in Freetown, Sierra Leone: A
- Current Networks of Long Proxies for Building Reconstruction Models of the Atlantic Multidecadal Osc
- Physicochemical Characteristics of Water Samples Collected from River Sokoto, Northwestern Nigeria
- Assessing the Impact of Climate Change on Maize (Zea mays) and Cassava (Manihot esculenta) Yields in
- Climatic Variations and Consumption of Urban Water
- Changes in Environmental Parameters and Their Impact on Forest Growth in Northern Eurasia
- Weather Impact on Heat-Related Illness in a Tropical City State, Singapore
